Copper clad laminates used in the microwave Printed Circuit Board (PCB) industry have many properties of concern. The dielectric constant (relative permittivity or εr) is one of the most critical. Many design engineers have made the assumption that this property is a rigid value; however, often that is not the case. The suppliers of copper clad laminates give the εr value on the product datasheet with accompanying information regarding the test method and testing frequency. One confounding issue is that the εr value of a particular material using one test method may not be the same value when testing the exact same material while using a different test method. Another concern is by the nature of the microwave circuit design, the fields within the material could be significantly different as compared to the method used to generate the εr value and this could give unexpected results as well.
This article will give an overview of the most common test methods used to determine the er value for microwave laminates. The limits and capabilities of these tests will be demonstrated as well. Several other electrical characterization techniques will be discussed, which are typically employed by means of simple microwave circuit evaluations.
General Test Method and Considerations
In a very broad sense there are two different types of electrical characterization techniques for microwave PCB laminates; one is a method using resonance and the other is using transmission/reflection techniques. Resonance methods are typically more accurate for determining εr; however, they are limited to a specific frequency or a few discrete frequencies. The transmission/reflection methods usually yield results over a range of frequencies. Both of these general test procedures are usually done in frequency domain; however, there are derivatives of both using time domain. In this article, frequency domain techniques will be considered.
Understanding electromagnetic field orientations within the material under test can be very important in assessing how applicable the resultant εr value from a test method is related to a given microwave application. For example, assuming a test method that generated an εr value within the x-y plane or the length-width plane of the material and not the z-axis (thickness) and if the microwave application has fields that are dominant in the z-axis, then the given εr value may have significant accuracy issues for that particular application. The significance is really related to several factors, some of which are the sensitivity of the circuit application as well as the anisotropic εr nature of the laminate. A laminate with higher anisotropy will have a larger difference in εr when comparing values of the x-y plane to the z-axis. It is common for laminates used in the PCB industry to have some level of anisotropy. Sometimes this is due to layers of glass fiber within the laminate used for mechanical stabilization.
Common Test Methods to Determine εr
There are typically two test methods that are most often employed by the laminate suppliers to determine dielectric constant. Both of these test methods are defined in the IPC-TM-650 2.5.5.1

Figure 1 Simple drawing of the X-band clamped stripline resonator test.
The first method discussed uses a stripline resonator and is intended to determine the z-axis εr value of the material under test. The method is tailored toward the testing of raw laminate in a high volume manufacturing environment. Because of the design of this test method, the εr value may or may not apply to a microwave application. The test procedure is per IPC-TM-650 2.5.5.5c and can be found at www.ipc.org. Essentially, the procedure is to have two samples of the material to be tested placed on both sides of a thin resonator circuit and have two ground plates apply pressure from both sides. The outer clamping plates act as the ground planes for the stripline structure, with the resonator circuit pattern in the middle and the material under test making up the dielectric layers (shown in Figure 1).

Figure 2 Broadband frequency response of X-band clamped stripline resonator: (a) and isolated resonant frequency peak at approximately 10 GHz (b).
The circuit pattern is designed to have the resonator element with a physical length of two wavelengths at 10 GHz, given a target εr value and a defined dielectric thickness. This test method is capable of determining the εr value and the dissipation factor of the material under test at frequency intervals of ½ wavelengths up to about 12.5 GHz. Typically, the resonant peak at 10 GHz is evaluated; this is shown in the detailed view of Figure 2b.
There are two potential issues for this test method. One is that some amount of air can be entrapped with the material under test and the method reports a lower than expected εr value. This issue is exaggerated when a laminate using a high profile copper is tested, due to the samples having more surface area when the copper is removed prior to the test. The second potential issue is the anisotropy effects of the material under test. The resonator circuit design is purposely loosely coupled in order to realize the Q of the material more so than the Q of the overall resonator circuit. The coupling is done by a gap coupling on the resonator circuit pattern and in the gap areas there is higher concentration of fields. It is in these areas where the anisotropy effects of the laminate can alter the center frequency of the resonator and cause a potential difference in the εr calculation. The fields in the gap area utilize the x-y plane properties of the material under test, whereas the element of the resonator is generally using the z-axis of the material. The calculation for εr for this test method follows:

where n is the resonant frequency node, c is the speed of light in a vacuum, fr is the resonant frequency, L is the physical length of the resonator element and ΔL is the added length in the gap coupled areas for the effects of electric field fringing.

Figure 3 Basic illustration of the full sheet resonance test method.
The second common test method used in determining the εr of a high frequency laminate is the Full Sheet Resonance (FSR) test method. This is also defined by an IPC test method: IPC-TM-650 2.5.5.6. This method is a non-destructive test as opposed to the stripline test, which requires the sample to have the copper etched completely off prior to testing the sample. The FSR test method determines the εr value of the laminate in the z-axis; however, it does not determine the dissipation factor. The method basically uses the copper clad laminate under test as an open walled parallel plate waveguide and evaluates an established standing wave. A simple drawing to illustrate the test set up is shown in Figure 3.

Figure 4 Broadband frequency response of a panel under test using the FSR test method.
From the resonant frequency peak of a standing wave, the er of the laminate can be determined. As with many resonator test methods, there are several resonant peaks to evaluate. However, most will be at a relatively low frequency for this test. A standing wave frequency peak and specifically the associated wavelength are directly related to the physical size of the panel. Most panels under test are 24" × 18" in size; therefore, the first dominate mode resonant peak will occur on the length axis of the panel and will be a long wavelength and thus a low frequency. It is common to have the first few measureable resonant peaks to be in the range of 100 to 300 MHz and a broadband image of a panel under test is shown in Figure 4.
The FSR test method does not have the issue of the entrapped air or anisotropy effects mentioned for the clamped stripline test; however, it does have limits. The low frequency testing will be less sensitive to some high frequency effects on determining εr. One such effect is related to the copper surface roughness of the laminate, where it was found that the propagation constant can be affected by the copper roughness and therefore the apparent εr value is affected as well.2
The simple calculation for the εr value of a panel under test using the FSR method is given:

where c is the speed of light in a vacuum, fr is the resonant frequency, and m and n are the resonant frequency nodes that relate to physical length (L) and width (W), respectively.
There are other common test methods used to evaluate the εr value of a PCB laminate and one that has become more popular lately is the Split Post Dielectric Resonator (SPDR) test method. This method uses perturbation methodologies with comparing resonant frequency peaks of the empty resonator verses a loaded resonator. The loaded resonator is a sample of the raw laminate with the copper fully removed. The SPDR test evaluates the εr value of the sample under test in the x-y plane only. This is a fast and user friendly test; however, it has a limit of the accuracy for the er value being directly related to the accuracy of the thickness measurement of the sample.
It has been suggested that a good general indicator for anisotropy of a laminate is to use the combination of the SPDR test and either the clamped stripline test or the FSR test. The SPDR test will evaluate the εr value of the laminate in the x-y plane, whereas the other two tests evaluate the z-axis of the material.
Common Microwave Circuit Evaluations for Determining εr
There have been a multitude of techniques defined to determine the εr value of a laminate by means of microwave circuit evaluations. Typically, a circuit will be designed and modeled for a very specific response, with the assumption of a particular εr value of the laminate, while trying to minimize all other effects on the circuit performance.
Three methods will be discussed here that utilize relatively simple microwave circuitry and have been proven to yield accurate results for εr characterization of a laminate. Due to the depth of information regarding these methods, the first will be discussed in an introductory manner with the appropriate references given for further investigation by the interested reader; the other two will be given with more functional detail.
A test method has been defined that uses a special microstrip resonator circuit to evaluate the εr values of a laminate in the x-y plane as well as the z-axis. The method was developed by Sonnet Software Inc. and its procedure and supporting data is explained in detail with several papers.3-5 The special resonator is a long edge coupled microstrip dual-mode resonator known as a RA Resonator. The circuit is relatively simple to fabricate and offers an accurate means to acquire the εr values of a laminate in multiple planes while using the same test circuit.
The next test method uses a pair of microstrip circuits to generate a relatively accurate εr value at several discrete frequencies. A simple gap coupled microstrip resonator has been used for many years with a few concerns. The major concern has been related to the gap coupling. The resonator should be loosely coupled to better realize the Q of the material, in order to get a more accurate determination of the εr value. If the resonator is too loosely coupled, the resonant peak can be distorted and a good measurement compromised. If it is not loosely coupled enough, the resonant peak may shift in frequency and cause the calculation of the εr to have some error. Also, since the gap coupling is a microstrip discontinuity, there is a concern for radiation losses adversely affecting the determination of the dissipation factor. With the following technique, the gap coupling concerns have been addressed.

Figure 5 Drawing of top view for two microstrip gap coupled resonators of different lengths.
Two microstrip gap coupled resonators are made on the same substrate and within very near proximity of each other. The resonators should be the same in every way, with the only exception being the resonator element length. One circuit should be significantly longer than the other, as shown in Figure 5.
To determine the effective er value of one of these resonators (1) is to be used and in that formula ΔL, is the added length of the resonator due to fringe effects in the gap area. With two resonators of different lengths using the same gap (ΔL), the effects of the gap can be eliminated. This is done by rewriting (1) for each of the two resonators, rearranging terms and finally solving the ΔL item to be eliminated follows:

Since ΔL has a small dependence on frequency, it is desired that f1 and f2 are relatively close in value. This is typically done by designing the resonator lengths to be integral multiples of each other and measuring higher order nodes of resonance. Back calculating the εr value from the effective εr has been done with the use of close form equations from Hammerstad and Jenson6 or field solving techniques.
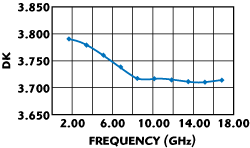
Figure 6 Results from testing two microstrip gap coupled resonators.
This test method is further described in a book7 regarding electrical characterization techniques of high frequency materials and a plot of results when using a common high frequency laminate is shown in Figure 6.
All the test methods described thus far have been resonator methods. The next test method is intended to determine the εr value of a laminate over a wide range of frequencies and using a transmission/reflection technique. This method also uses the advantage of having two microstrip circuits built on the same material and eliminating some variables that are common to evaluating this type of circuit. The circuit is a simple single-ended microstrip transmission line having two circuits of significantly different lengths. The procedure is detailed in a paper8 and the following is an overview.
Two circuits are made on the same substrate and very near in proximity to each other. Both circuits should be identical in every manner with the exception of length. One circuit should be significantly longer than the other by a multiple of three or more. Both circuits should use the same connectors for testing or ideally the same fixture. A measurement is to be taken for each circuit over a range of frequencies to obtain the phase angle at each frequency. The microstrip transmission line phase response formula is used to calculate the effective er value at each frequency. The phase response formula used is:

where Φ is the phase angle, f is the frequency, Eff_εr is the effective dielectric constant, c is the speed of light in a vacuum and L is the length of the transmission line. This is for a phase angle measurement at a specific frequency and a discrete transmission line length.
This method uses the two different transmission line lengths (ΔL) and their different phase angle (ΔΦ) at a specific frequency to determine the effective εr. The frequency is then incremented, the new phase angles accounted for, the effective εr determined and then recalculated at the new frequency.

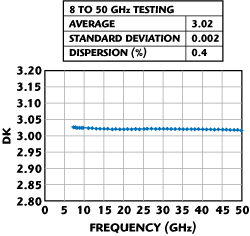
Figure 7 Results of the microstrip differential phase length test method.
At each point in the iteration process where the effective εr value is determined, a computer routine is used to back calculate the εr value of the laminate, as previously described. A plot of two circuits tested with this procedure using a common microwave laminate is shown in Figure 7.
Several different test methods for evaluating εr for microwave PCB laminates have been shown. Different test methods using microwave circuit characterization have been shown as well. Each of these methods have their own set of capabilities and limits that need to be understood in order to appreciate the significance of the εr value reported for a laminate in regards to a particular application.
It is strongly recommended for the designer to evaluate and define an appropriate test method that will best approximate their actual circuit application. The εr values supplied by the laminate manufacturers should be considered approximate in an effort to support the circuit designer for fine tuning their application with a material under consideration.
References
- IPC-TM-650 Standard Test Methods, www.ipc.org.
- J.W. Reynolds, P.A. LaFrance, J.C. Rautio and A.F. Horn III, “Effect of Conductor Profile on the Insertion Loss, Propagation Constant, and Dispersion in Thin High Frequency Transmission Lines,” DesignCon 2010.
- J.C. Rautio, “Measurement of Planar Substrate Uniaxial Anisotropy,” IEEE Transactions on Microwave Theory and Techniques, Vol. 57, No. 10, October 2009.
- J.C. Rautio, “A Proposed Uniaxial Anisotropic Dielectric Measurement Technique,” IEEE MTT-S International Microwave Symposium Digest, Guadalajara, Mexico, February 19-20, 2009, pp. 59-62.
- J.C. Rautio, “Measurement of Uniaxial Anisotropy in Rogers RO3010 Substrate Material,” COMCAS 2009.
- E. Hammerstad and O. Jenson, “Accurate Models of Microstrip Computer Aided Design,” 1980 MTT-S International Microwave Symposium Digest, May 1980, pp. 407-409.
- L.F. Chen, C.K. Ong, C.P. Neo, V.V. Varadan and V.K. Varadan, Microwave Electronics, Measurement and Materials Characterization, John Wiley & Sons Ltd., 2004.
- N.K. Das, S.M. Voda and D.M. Pozar, “Two Methods for the Measurement of Substrate Dielectric Constant,” IEEE Transactions on Microwave Theory and Techniques, Vol. MTT-35, No. 7, July 1987.
RO3010 is a licensed trademark of Rogers Corp.
