Frequency converters, a key component in modern satellite communications systems, create special characterization challenges for developers and manufacturers. The signals, processed by these converters and their modulation formats, can change dramatically from day-to-day and even hour-to-hour. The channels may be re-allocated to different services, and with its high cost, the life of the satellite may be expected to last longer than the services for which it was initially launched.
Because of these factors, frequency converters must be tested with essentially generic signals and, from these simple tests, the distortion effects on more complex signals can be adequately predicted. One of the key tests is the frequency response across the conversion channels, including amplitude, phase and group delay ripple. While the amplitude response is relatively easy to measure, the phase and especially the group delay response of satellite converters present particularly difficult challenges. These difficulties often lie in the fact that, unlike most other applications of converters, the local oscillators (LO) are embedded in the converter and are completely inaccessible to outside instruments, even to the point of not providing a common 10 MHz reference signal.
A technique was previously developed that provides for an absolute group delay measurement,1,2 but this method requires a second reference mixer to provide an IF phase reference, and until now, it also required an additional LO signal identical to the LO provided to the device under test (DUT).
Now a new method has been developed, applying that technique to the case of a frequency converter with an embedded LO. The difficulties in this technique (that is if there is no common reference) are described by Ballo.2 The key aspect of this technique is frequency tracking the IF of the DUT, such that the frequency of the external LO used for the reference channel mixer can be adjusted to accommodate offset and drift in the DUT embedded LO. Further, the phase of the IF of the DUT is also tracked to accommodate phase shift or slight frequency offsets (less than 1 Hz offset is required to avoid difficulties in the delay measurement). This tracking is done through software techniques, which do not require additional phase-locking hardware or added pilot tones.
Understanding Effects of an Embedded LO
To evaluate the effects of an embedded LO on a mixer or frequency converter, it is important to review some
details of the method.2 The group delay of the frequency converter is computed from the phase response measurement of the converter. Normally, the group delay is defined as 
where
φ(ω) = phase of the frequency response
ω = radian frequency
For a mixer, an equivalent S-parameter representation has been described by Williams, et al,3 which takes into account the effects of the phase of the LO, and defines the scattered waves as those scattered at the input, at the input frequency, and those scattered at the output, at the output frequency. The phase response of a mixer is given by3 
where φM is the phase response of the mixer (here ω is taken as the input frequency, but the assignment is arbitrary; aLO represents the LO drive signal, where the magnitude is assigned a unity value, so this essentially represents the phase of the LO; a1(ω) is the input signal at the RF (input frequency), and b2(ω – ωLO) is the IF (output) signal at the output frequency. By rearranging Equation 2, the output phase related to the input is found to be 
where φ denotes taking the phase of the wave. In the case of a mixer with a constant LO, the effect of LO phase on the group delay is inconsequential. In cases where the LO is not constant, but accessible, a sample of the LO can be used to drive the reference mixer, effectively eliminating errors due to drift. However, in the case of an embedded LO, where the LO is not available to the reference channel, phase and frequency drift versus time affect the measured phase result at the output. When this drift is significant with respect to the measurement time aperture of the measurement system, it will have an effect on the group delay response. The effect of LO phase noise and drift can be illustrated by an example.
Consider a measurement with an IF bandwidth (BW) of 3 kHz and a measurement time of approximately 1/3 kHz or 330 μs. Two measurements are made at 100 kHz RF spacing, with 50 μs settling between points, thus the average measurement time is 380 μs (here one-half of each measurement time represents the average of the LO frequency change over that time). For the sake of this example, assume an LO frequency of 20 GHz that drifts at 0.1 ppm/day, which results in a drift of 2 kHz/day or 0.023 cycles/second or 8.33° per second. Over the measurement time-window, the LO would drift approximately 0.003°, which would affect the group delay by 0.003/(360 x 100 kHz) = 83 ps. This delay, due to drift of the LO, is in addition to any error from the LO being off frequency from the LO used in the reference channel mixer. The delay error due to the frequency error can be computed as
Consider a frequency offset error of 1 Hz. This represents 360°/sec, for a delay error of 3.8 ns, again assuming that the measurement time is 380 μs and the group delay frequency aperture is 100 kHz. Further, if the LO has a phase noise, it will directly translate to the IF phase, though the conversion of phase noise to phase deviation is not easily determined. One estimate is to assume the phase noise at the offset equivalent to the IF filter can be converted to phase deviation by treating it as an added noise floor. For example, at a 30 kHz offset, the phase noise might be –60 dBc, which may appear as approximately 58 milli-degrees of trace noise on the phase trace, and 1.5 ns of group delay noise. In many cases, a much lower level of delay noise is desired. In other circumstances, averaging is used to reduce the noise in a measurement, but without further processing, averaging will not work on these devices.
Details of the Measurement System
The measurement system is shown in Figure 1. Note that the DUT is a conversion chain of any number of LOs. The calibration of the measurement system proceeds as described by Ballo.2 For the measurement of the DUT, however, the LO of the DUT is used instead of the external LO. To avoid errors associated with offset in the DUT LO frequency, it must be measured very precisely.
The LO frequency is determined in two steps: first a broadband frequency sweep may be made to determine the approximate frequency; a phase versus time sweep may then be made to determine the precise frequency. For many converters the approximate frequency is known, so the frequency only must sweep a narrow bandwidth, which may be set by the user. In practice, the VNA first IF (approximately 8 MHz) sets the maximum span of the broadband sweep, since going wider than about twice the IF may cause an image of the signal to appear in the receiver. The resolution of the broadband sweep is determined by both the number of measurement points and the bandwidth selected so more points are required for wider sweeps.
Figure 2 shows the result of a broadband sweep, in which the magnitude of the IF output from the DUT is slightly off the center frequency (about 60 kHz high), where the frequency resolution is 10 kHz. Next, the reference LO and the receiver frequencies are offset by 60 kHz, and the measurement is switched to a ratio measurement to compare the phase of the DUT output with the phase of the reference mixer output, as a function of time. Since a digital IF is used in modern VNAs, phase versus time is only valid as long as the frequency offset from the VNA center frequency is less than one-half the IF BW (the IF BW sets the sampling rate). Because of this issue, the broadband sweep automatically sets the number of points so that the resolution is less than one-third the tuning IF bandwidth (BW). If the frequency is known more precisely than the tuning IF BW, then there is no need to use broadband tuning, which will improve the measurement time. Figure 3 shows the first phase versus time plot. From this, the frequency error can be computed as
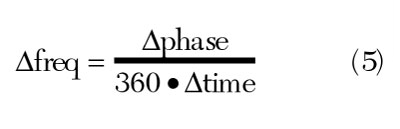
For the sweep time of 2.83 ms, the offset is approximately 1 Hz per degree. This is a remarkably precise measurement of the frequency offset, and it can be done in a very short time. After this pass, the offset is just over 1 kHz (the approximation from the first step shows the external LO is about –59.985 kHz low). The frequency is reset to this value, but the IF filter has some phase response so an additional iteration is needed. Figure 4 shows the result of the next phase versus time plot. With an offset of +31°, the LO frequency is –59.016 kHz.
A final measurement phase versus time has almost no phase slope and the overall CW phase versus time trace has approximately a 1° of peak-to-peak noise, but using a least squares fit of the entire trace allows a precise computation of the average offset value over the measurement time, for a final value of –59,015.9 Hz low. Notice that with these four sweeps, a precise alignment of the reference LO with the external DUT LO is achieved with a total measurement time of less than 20 ms.
The tuning sweep can be performed as often as just before every sweep to determine the frequency drift. For cases where the embedded LO is quite stable, the tuning may not need to be done on every sweep; the update rate can be set by the user, as shown in the user-interface dialog of Figure 5. Now that the reference LO is frequency aligned with the embedded LO, the VNA can make a normal group delay measurement, but effects of the phase noise of the LO will still present an obstacle.
Phase Locking the Result
Now the system is set to measure the phase versus frequency of the DUT converter over the measurement frequency span. However, the frequency is still not completely precise, and the error frequency will cause the phase of the response to precess over time. Figure 6 represents a polar view of a single frequency vector response measured over several sweeps (solid blue vectors). The average of these signals (dotted vectors) will tend toward zero as the phase varies sweep-to-sweep.
Figure 7 shows the delay response without averaging in two cases: the upper (memory) delay trace shows the delay when a common LO is used for both the reference and test mixers; the middle delay trace shows the response using two separate LOs, but locking the 10 MHz reference together. The noise on the delay trace for the locked LOs is approximately 2.5 times greater than that of a shared LO (where phase noise effects are canceled). In both cases, 20 averages were used to reduce noise. The blue trace shows the amplitude response of the mixer. Figure 8 shows the result with averaging, when the LOs are not locked to the same reference. The delay trace is about 25 times noisier, and the magnitude response is reduced due to the averaging effect. In this case, the phase of the signal to be averaged varies by a random amount each sweep. Thus, when this response is averaged, the signal level of the averaged trace is reduced, and with a constant noise floor, the noise begins to dominate. This is the result typically seen using the methods described in Reference 1.
An added process step in the new method resolves this problem by phase tracking the response of each sweep within the software, and using the measured phase tracking to compensate the overall response to maintain a constant phase, at a frequency selected by the user. Figure 9 compares the new method of software phase tracking with a measurement using a shared LO. The results are indistinguishable from those using a locked 10 MHz reference. Here the nearly identical group delay trace noise demonstrates that the software phase tracking technique provides equivalent frequency and phase stability to locking the time-bases of two independent oscillators. Note that the noise in the measurement is less than 125 ps rms, which is the limit of the measurement quality.
With this new method, the full error correction capabilities of a vector network analyzer can now be applied, including correcting for input and output mismatch, to a new class of devices: frequency converters with embedded local oscillators. The method has been applied to high gain converters, and provides good phase tracking when the signal-to-noise (measured with a 2 MHz resolution BW) is as little as 10 dBc. With frequency tracking and software phase locking, a remarkably accurate measurement of the group delay of these devices of less than 100 ps can be achieved.
References
1. J. Dunsmore, “Novel Method for Vector Mixer Characterization and Mixer Test System Vector Error Correction,” 2002 IEEE MTT-S International Microwave Symposium Digest, Vol. 3, pp. 1833–1836.
2. D. Ballo, “Measuring Absolute Group Delay in Multistage Converters,” 2003 European Microwave Conference Digest, pp. 89–92.
3. D.F. Williams, F. Ndagijimana, K.A. Remley, J. Dunsmore and S. Hubert, “Scattering-parameter Models and Representations for Microwave Mixers,” IEEE Transactions on Microwave Theory and Techniques. Vol. 53, No. 1, January 2005, pp. 314–321.
Joel Dunsmore received his BSEE and MSEE degrees from Oregon University in 1982 and 1983, respectively. Since then he has worked for Agilent Technologies Inc. (formerly Hewlett Packard) in Santa Rosa, CA. He received his PhD degree from Leeds University, UK, in 2004. He was a senior design contributor working in the Component Test Division and a principal contributor to the HP 8753 and PNA family of network analyzers, responsible for RF and microwave circuit designs in these products. Recently, he has worked in the area of nonlinear test, including differential devices and mixer measurements.
