To calculate the loaded Q (Q_L) consider the unloaded Q (Q₀) and the loading effect of the transistor. There we consider the influence of the large signal condition Y₂₁*. The inverse of this is responsible for the loading and reduction of Q_L.

The transistor noise factor can also be calculated under large signal conditions. Considering Y₂₁*, this noise calculation is based on general noise calculations such as those of Pucel and Rohde.¹⁰ An equivalent procedure can be found for FETs as well.

Oscillator Noise Factor

Figure 4 shows the oscillator equivalent circuit configuration for the purpose of analyzing F with respect to the oscillator feedback components (C₁ and C₂), which are needed to predict F in Leeson’s Equation. The objective is to determine the oscillator circuit parameters that influence F, thereby influencing oscillator phase noise.

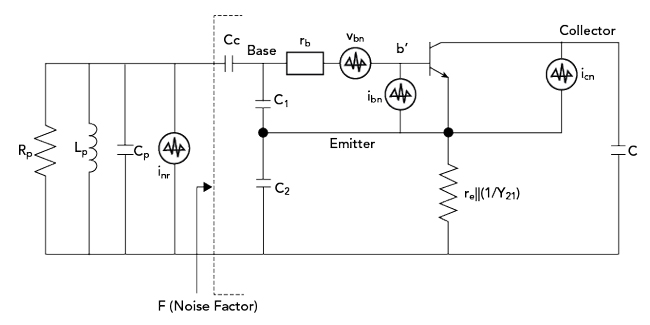
Figure 4 Colpitts oscillator circuit configuration.

F for the transistor in Figure 4 is:

For fixed capacitors C₁, C₂, the following components contribute to oscillator noise:

• Thermal noise associated with the loss resistance of the resonator
• Thermal noise associated with the base resistance of the transistor
• Shot noise associated with the base bias current
• Shot noise associated with the collector bias current.

An oscillator circuit with the contribution of all four noise sources is shown in Figure 5.

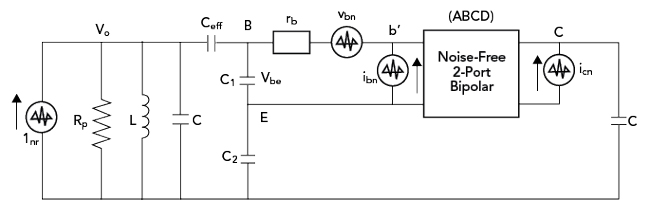
Figure 5 Oscillator calculation using the ABCD matrix.^{3, p. 132}

Using an oscillator circuit with a noisy resonator, the total noise of the oscillator in Figure 5 can be determined.³ Consider the effective capacitance C_eff:

The phase noise contribution (PN) from the oscillator circuit with a 2-port [ABCD] matrix is:

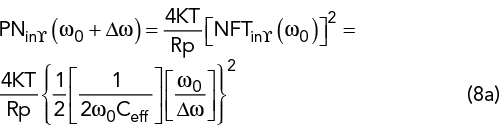
= the phase noise contribution from the resonator tank.

= the phase noise contribution from the base spreading resistance.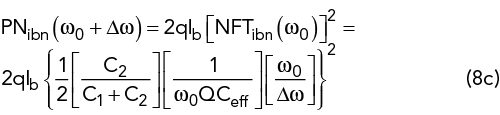

= the phase noise contribution from the base current.

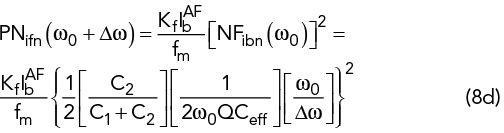
= the phase noise contribution from the flicker noise of the transistor.

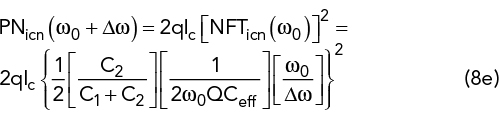
= the phase noise contribution from the collector current.

AM-to-PM Conversion

The transition from a basic oscillator to a VCO is achieved by replacing all or a part of the resonator capacitor with a voltage dependent variable capacitor, typically a tuning diode or varactor.² The RF voltage across the depletion capacitance modulates the operating frequency. FM and PM cannot be distinguished at a constant f_m; however, with a change in f_m, the FM modulation index will change and the PM modulation index will not.

To calculate the total phase noise, an equivalent noise resistance R_eq of the tuning diode is defined. When inserted in Nyquist’s equation it results in a frequency offset dependent noise voltage V_n across the tuning diode:

where kT = 4.2 × 10×^-21 at 300 K, and Δf is the offset from the carrier.

This noise voltage generated from the tuning diode is now multiplied with the VCO gain K_o, resulting in an rms frequency deviation Δf_rms. This can be translated into an equivalent peak phase deviation θ_d:

This is converted into the single sideband (SSB) signal-to-noise ratio expressed in dB

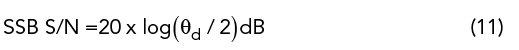
Comparing the SSB noise of the oscillator with a fixed capacitor and an identical capacitance value of the tuning diode determines the equivalent noise resistor R_eq. The lower the resistor the better the quality of the diode. All relevant information is provided by Rohde and Rudolph.⁹

DIODE FABRICATION AND SINGLE DEVICE PERFORMANCE

A Si tuning diode consists of a p-n junction. Contacting an n-doped and a p-doped region, the mobile charge carriers (electrons and holes) are pulled away from the junction and a depletion zone is created that acts as a capacitor. By applying a reverse bias voltage (V) the depletion zone width x_D increases. For the n-type region it is given by:

ε₀ε_Si is the dielectric constant of Si, e is the elementary charge and N_D(x) is the donor concentration. The diffusion voltage V_D is given by:

where W_c is the energy of the conduction band edge and W_F is the Fermi energy.

For the p-type region, the donor concentration is replaced by the acceptor concentration.

The voltage dependent capacity C(V) can be written as:

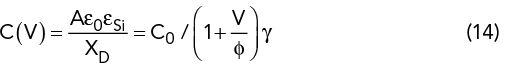
where γ = 0.5 characterizes the theoretical value for an abrupt junction diode with constant doping concentration in the depletion zone.

For a VCO, several more parameters must be considered, such as frequency range, linearity, series resistance and noise level. So-called hyperabrupt tuning diodes¹¹ are widely used for VCOs and this article presents results regarding these requirements. Using epitaxy, ion implantation and careful thermal management, high-quality hyperabrupt diodes are realized with extremely low noise levels.

Highly doped n-type 200 mm Si wafers with (100) orientation are used to achieve low series resistance. In a first step, an n-type epitaxial layer with a thickness of several micrometers and a doping concentration in the range of 1×10¹⁵ to 1×10¹⁶ cm^-3 is grown. The p⁺-region is fabricated by ion implantation.

A SIMS profile of the total stack after annealing is shown in Figure 6. In principle it is possible to create even sharper doping transitions,12 however, these techniques (e.g. molecular beam epitaxy) are not suited for mass production. To avoid lateral spreading of the electric field, a guard ring structure is integrated. Diodes with an active area of A = 0.118 mm² as well as A = 0.037 mm² are fabricated on the same wafer. For the front-side metallization a Ti/TiN barrier is sputtered followed by Al/1%Si. For the backside a gold-based metallization is chosen to enable soldering in the package.

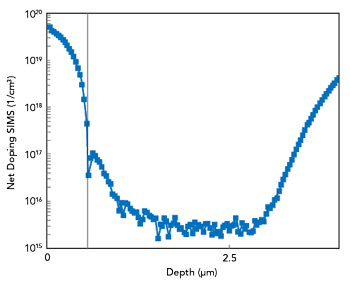
Figure 6 SIMS profile of the hyperabrupt tuning diode.

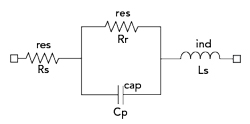
Figure 7 Diode equivalent circuit.

The noise of the hyperabrupt diodes—and therefore the resonator noise—depends on the quality factor Im(Z)/Re(Z). The higher the quality factor of the resonator, the lower the oscillator noise. Considering the equivalent circuit of the diode (see Figure 7), one must consider two parameters:

1) The crystalline quality of the Si in the depletion zone should be as high as possible to avoid any point defects such as vacancies or interstitials, which act as scattering centers and increase the noise level.¹³ As a consequence, the resistance R_r for reverse bias must be as high as possible. This can be achieved by careful annealing steps.