Electrical noise is created naturally by almost any type of device. In circuits that need to amplify and process very low level signals, noise can interfere with the desired signal, causing degraded or complete loss of reception. This is significant for any wireless receiver, often being the limiting factor on reception quality and range. Much of the interfering noise can be generated by the components in the receiver circuit itself.
There are two approaches that can reduce the effect of noise. The first is to increase the transmitted signal level so that the receiver noise becomes insignificant. However, this is usually not practical because of cost, power, size, weight, safety, or regulatory limitations. The other approach is to reduce the noise generated by the receiver circuitry. This is generally the only cost-effective and practical option available.

Figure 1 Noise figure is defined in terms of input and output signal-to-noise ratios.
Noise figure is a measure of noise generated by a circuit. It is defined as the input signal-to-noise ratio divided by the output signal-to-noise ratio. It can be expressed as a ratio, sometimes called noise factor, or in dB, as shown in Equation 1 and illustrated in Figure 1. For an ideal, noise-free device, the input and output signal-to-noise ratios would be equal, giving a noise figure ratio of one. In any real device, some noise is added, so the noise figure ratio is always greater than one. One of the objectives for circuit designers is to make the noise figure as low as possible to minimize the adverse effects of noise in the system.

At microwave frequencies, the primary type of noise is thermal noise, which is generated by any resistance in the circuit, or by the active devices used to amplify signals. Resistance is due to discrete resistors or the resistivity of lossy components. The available noise power produced by a resistance is given by Equation 2:

where
Pn = available noise power
k = Boltzmann’s constant
T = temperature in Kelvin
B = bandwidth of the system
An active device, such as a transistor, can be modeled as an ideal noise-free two-port network plus two noise sources, as shown in Figure 2. Generally, the two noise sources are partially correlated to one another, so their combined effect either adds or cancels, depending on the value of the source impedance driving the device, expressed in terms of source reflection coefficient, Γs. This means that the noise figure of the device will be a function of Γs. This function must be understood in order to design circuits for optimum noise performance.

Figure 2 Basic noise model of an active device.
Noise Parameters
The variation of noise figure versus Γs is described by Equation 3, commonly called the noise figure equation. The parameters Fmin, rn and Γopt are called the noise parameters, and they actually consist of four scalar values, because Γopt is complex and therefore has a magnitude and a phase component. If the noise parameters are known, then the circuits using the device can be designed to minimize the effects of noise.

where
F= noise figure ratio of the device
Γs = source reflection coefficient
Fmin= minimum noise figure
rn = normalized noise resistance
Γopt= optimum source reflection coefficient associated with Fmin
There are other forms of the noise parameters, such as different configurations of the noise correlation matrix, but this set is the most commonly used. If the S-parameters and noise parameters of a device are known, then converting from one form to another is straight forward, similar to converting between S-parameters and Y-parameters, for example.
Noise Parameter Measurements
The basic approach for determining the noise parameters is to measure the noise figure of the device-under-test (DUT) with four different known source reflection coefficients, and then use these values to solve four simultaneous noise-figure equations, yielding the values of the four scalar noise parameters. However, noise measurements tend to be sensitive to small errors, so in practice, more than four measurements are made, and then a least-means-squares algorithm is used to reduce the over-determined data.1,2
Another practical measurement improvement is the use of the noise power formulation shown in Equation 4, instead of the previously shown noise figure equation. This equation allows rigorous accounting of the reflection coefficient difference between the hot and cold states of the noise source. It also allows use of any combination of hot and cold measurements, including the cold-only measurement method commonly used by vector network analyzers.

where
P = total measured noise power
k = Boltzmann’s constant
B = system bandwidth
t0 = reference temperature of 290 Kelvin
tns = temperature of the noise source in Kelvin
F1 = device noise figure (a function of source reflection coefficient and device noise parameters)
F2 = measurement receiver noise figure (a function of the DUT output reflection coefficient and receiver noise parameters)
Ga1 = DUT available gain (a function of the source reflection coefficient and DUT S-parameters)
Gt2 = measurement receiver transducer gain (a function of the DUT output reflection coefficient, receiver S-parameters and receiver signal-processing gain)
Traditional Noise Parameter Measurements
A traditional noise parameter measurement setup is shown in Figure 3. The RF switches connect the DUT to either the network analyzer or the noise figure meter. The network analyzer is used to measure the S-parameters of the DUT with the tuner set to 50 Ω. The noise figure meter is used to measure noise power for any Γs set by the source tuner. When measuring devices that require bias through the RF ports, external bias tees are configured as shown so that the bias is independent of the switch position. An optional load tuner (not shown) is sometimes used with highly reflective devices to reduce sensitivity to error.
The system calibration includes measuring the tuner at many states that cover the whole Smith chart independently at every frequency. This allows the selection of a good set of Γs values at every measurement frequency, which is desirable because good measurement accuracy and sensitivity requires the correct selection of tuner impedance states. The reference planes of the tuning block must go from the noise source plane to the DUT input plane.

Figure 3 Traditional noise parameter measurement strip.
An in-situ system calibration can be done after doing two network analyzer calibrations. The first is a two-port calibration at the DUT planes. The second is a one-port calibration at the noise source port. By subtracting the error terms of the two calibrations, the two-port S-parameters from the noise source port to the DUT input port can be determined. If an optional load tuner is used, then an additional one-port calibration at the noise receiver plane will be used to get the S-parameters from the DUT output port to the noise receiver port.
To save time in the event that something outside of the tuner changes (a wafer probe, for example), the tuner may be characterized separately. A hybrid in-situ calibration can then be done in the same manner to get a fixed S-parameter block that does not include the tuner.
After the system calibration is complete, the noise receiver is calibrated one frequency at a time; the DUT noise parameters are then measured one frequency at a time.3,4 This is done because the noise parameter extraction involves complex calculations that are sensitive to small errors, so it is important to select a good pattern of source reflection coefficients to get well-conditioned data.2 Making the measurements one frequency at time allows an ideal pattern to be selected.
One problem with the traditional approach is that it is very time consuming, due to the large number of tuner states (each requiring physical movement of the probe/carriage assembly), and the large number of single-frequency noise measurements. It is common to sweep 400 or more frequency points in S-parameter measurements, but for measuring noise parameters, that many frequencies would take days. With long measurements, temperature drift can cause significant errors. This is exacerbated by the many lengths of cables required for all the instrument and component connections shown in the measurement setup.
Since traditional noise parameter measurements are slow, they are typically limited to a sparse set of frequencies. But this makes the scatter, outliers and cyclical-frequency errors difficult to interpret. A cyclical error is common with imperfect network analyzer calibrations, where the system errors will add at some frequencies and cancel at others. This can cause an aliasing effect, which can shift the data values up or down. Smoothing techniques can make the data look better, but will not correct for this type of data shift.
New Ultra-Fast Noise Parameter Measurements
The new ultra-fast noise parameter measurement method (patent pending) typically speeds up measurements by over two orders of magnitude. This is a major breakthrough for the industry, and makes much larger frequency sets practical.
There are two key features of the new method that contribute to the large speed improvement. The first is that the tuner is characterized with a single set of tuner states (physical tuner positions), each of which is swept versus frequency. The tuner’s states are selected to give a good spread on the Smith chart at every frequency within the selected frequency band. The second feature is that the noise power data is swept over frequency, one tuner position at a time. The tuner only has to move to each position once, and the measurements take advantage of the fast sweep times of modern instruments. The fast measurement time virtually eliminates temperature drift as a source of error.

Figure 4 New noise parameter measurement setup.
The new method has been implemented with Agilent’s PNA-X network analyzer, which has an optional built-in low-noise receiver and a flexible, switched test set, which greatly simplifies the measurement system, as shown by the block diagram in Figure 4. This reduces the number of cables and connections, helping to stabilize the setup, and offers fewer opportunities for error. A photograph of the setup is shown in Figure 5. The Maury tuner is controlled by a USB cable plugged into the front of the PNA-X.

Figure 5 Photograph of the new setup measuring a coaxial DUT.
Since a fixed set of tuner states is used for the entire frequency band, the reflection coefficient pattern may not be as ideal at some frequencies as with the traditional method, depending on how the states are selected. For example, Figure 6 shows the result of selecting uniformly spaced mechanical tuner states. The pattern is fine at one frequency (left chart), but very poor at another frequency (right chart).

Figure 6 Aliasing due to uniform spacing of tuner states results in poor patterns at some frequencies.

Figure 7 Non-uniform spacing of tuner states provides good patterns across frequency.
A solution to this is to use non-uniform spacing of tuner states. Figure 7 shows the result at the low, middle and high end of the band. As the frequency varies, the points rotate, but a good pattern is maintained at all frequencies. The pattern does use about 50 percent more points than typically used in a traditional noise parameter measurement, but that still allows two orders of magnitude speed improvement.
The new method may be done in multiple frequency bands with tuners that have frequency-banded mismatch probes. For the examples in this article, the data was taken with a Maury tuner model MT982EU30. The low frequency mismatch probe covered 0.8 to 2.8 GHz; the high frequency mismatch probe covered 2.8 to 8.0 GHz. The noise software installs and runs inside the PNA-X itself, although it can also be run from a separate computer.
Comparison of the Two Methods
To test and compare the two methods, a microwave FET was permanently mounted in a stripline fixture with 3.5 mm connectors as seen in the photograph of the new system, and the connector planes were the DUT measurement planes. This produced the repeatable connection required for a good comparison. The measurement covered 0.8 to 8.0 GHz, with steps of 0.1 GHz, resulting in 73 frequencies. No smoothing was applied to any of the data shown here.
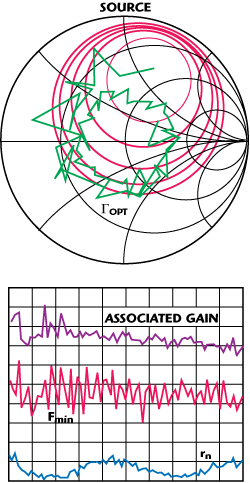
Figure 8 Noise parameter data from traditional method with 73 frequencies.

Figure 9 Same data as Figure 8 using only 15 frequencies, giving misleading results.
Figure 8 shows the measured data using the traditional measurement method. Fmin is approximately 1 dB, and fairly flat versus frequency. Fmin scatter is about ± 0.2 dB at the lower frequencies, improving to about ± 0.1 dB at the higher frequencies. The scatter is quite uniform, so smoothing would probably be quite effective. But the measurement took 30 hours, 15 minutes, from start to finish.
This scatter is typically not observed by RF designers, since measuring 73 frequencies is actually unrealistic for the traditional method, since it takes too long. Measuring with a step size of 0.5 GHz would be more typical. Figure 9 is exactly the same data, except that all the frequency points in between a 0.5 GHz spacing were deleted, leaving only 15 frequencies, which is typical for the traditional method. The data looks fairly smooth, but there is a very odd hump in the frequency response of Fmin. It appears that something strange is going on with the DUT with the shifted Fmin data. But if it is compared to the original data, it is seen that the DUT is normal, and the strange hump is due to aliasing of the data, where low points of the scatter are picked over part of the frequency range, and high points of the scatter are picked over a different part of the band. The dense frequency spacing shows a much more accurate data, and smoothing techniques also become more meaningful with the large number of points.

Figure 10 Noise parameter data taken by the new method.
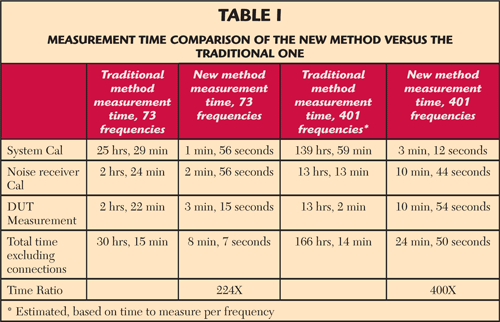
Figure 10 shows the measured data taken with the new method, using the same 73 frequencies. Again, no smoothing is applied to the data, but the scatter is much smaller, so smoothing is not necessary anyway. Using the new method, the measurement took only eight minutes, improving the test time by a factor of 224. A time comparison is shown in Table 1 for 73 and 401 frequencies.
Another significant benefit of the new method is the simplicity of the test setup, calibration and measurement procedure. The connections and calibration process is now similar to that required for S-parameter measurements, so instead of requiring a highly skilled engineer, a technician or other trained operator can easily accomplish the tasks.
Improved Accuracy
The primary motivation for this work was to speed up and simplify the measurement. But a side benefit is improved accuracy. Some reasons why this makes sense include:
- The new method uses a simpler setup. There are fewer cables and connections, and therefore fewer opportunities for problems from cable movement, loose connectors, etc.
- With the new method, a full in-situ calibration is always performed, because it is so fast. This removes the accumulated errors of multiple S-parameter calibrations, and eliminates reconnection errors.
- With the new method, there is minimal drift due to the short calibration and measurement times.
- With the new method, a dense frequency selection is used to eliminate aliasing, because it is fast.
- Smoothing was not shown in any of the data here, but the dense frequency selection would make smoothing more meaningful.
Opportunity for Production Testing
Production testing of noise parameters has never been an option with the traditional measurement method because of long measurement times. However, the speed and simplicity of the new method opens up new possibilities in manufacturing applications. Production testing of known parts may not require many frequencies, so the measurement time for each device could be less than 30 seconds. Providing noise-parameter specifications or measured data for each part makes products more competitive and more valuable to customers. Circuit designers can use the noise parameters to better predict noise performance of devices in mismatched environments, or to design better matching circuits. In addition, test data allows manufacturers to sort for performance, and to track production runs for continuous process improvement, providing an overall higher level of quality assurance.
Conclusion
The new ultra-fast noise parameter measurement method provides more than two orders of magnitude speed improvement, and more accurate data with a simpler setup that does not require a highly skilled operator. The fast measurements virtually eliminate drift, greatly reduce measurement scatter, and allow dense frequency spacing, providing more accurate and more complete data, and better insight into device noise performance.
Acknowledgments
The authors thank Lynn Rhymes of Agilent Technologies Inc., Santa Rosa, CA, for supplying the connectorized test device.
References
1. R.Q. Lane, "The Determination of Device Noise Parameters," Proceedings of the IEEE, Vol. 57, No. 8, August 1969, pp. 461-1462.
2. C. Giuseppe and M. Sannino, "Computer-aided Determination of Microwave Two-port Noise Parameters," IEEE Transactions on Microwave Theory and Techniques, Vol. 26, No. 9, September 1978, pp. 639-642.
3. "Operating Manual, Automated Tuner System," Maury Microwave Corp., Document number MT993-2.
4. R.Q. Lane, "A 0.5 to 18 GHz Semi-automatic Noise Parameter Measurement Technique," 19th ARFTG Digest, June 1982, pp. 42-58.
5. G. Simpson, D. Ballo, J. Dunsmore and A. Ganwani, "A New Noise Parameter Measurement Method Results in More than 100x Speed Improvement and Enhanced Measurement Accuracy," 72nd ARFTG Digest, December 2008.
