Figure 6 compares the reflection coefficients of the different materials versus incident angle at selected frequencies. The reflection coefficient of the mirror and glass is generally higher than that of plaster and wood. In addition, all four materials follow the rule that the reflection coefficient increases with the incident angle, which is consistent with the characteristics of vertically polarized waves.





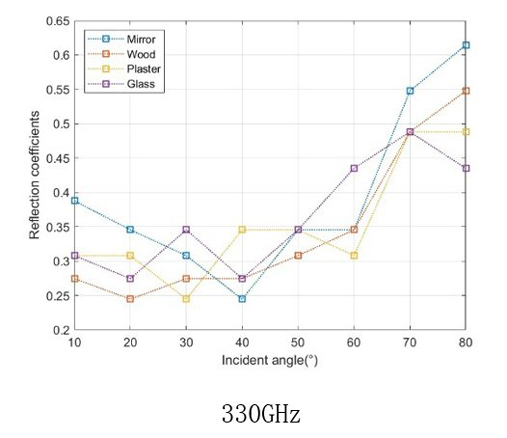
Figure 6 Measured reflection coefficients of four materials as a function of incident angle at 240 (a), 250 (b), 280 (c), 290 (d), 320 (e) and 330 (f) GHz.
Note that there are local deviations from the overall trend. It is possible that this is an error in the experimental process, which may be a consequence of the more complex factors to be considered in the reflection path and power measurements compared to the LOS path. However, we prefer to believe that there are other factors contributing to the fluctuations in the data, such as the complex permittivities, which must be verified by more refined and extensive experiments in the future.
Figure 7 shows the reflection coefficients of the different materials over frequency at an incident angle of 80 degrees. When the incident angle is constant, the reflection coefficient decreases as frequency increases. Generally, the Rayleigh criterion is used to characterize the roughness of a medium surface relative to the wavelength of incident light:18
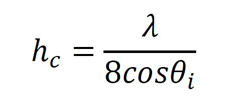
According to the Rayleigh roughness coefficient theory, when the incident angle is constant, higher frequencies are more likely to be considered rough on the same surface. The experimental results fit well with this theory.

Figure 7 Measured reflection coefficient of different materials as a function of frequency at a fixed incident angle of 80 degrees.
MMSE Method for Estimating the Dielectric Constant of Reflective Surfaces
In the theoretical model, there are four variables that affect the change in the reflection coefficient, namely incident angle θi, wavelength λ, relative dielectric constant εr and surface roughness hrms. The values of θi and λ can be easily determined, but εr and hrms are difficult to measure; so, it is difficult to evaluate the accuracy of the model by comparing the theoretical and measured values. However, the process can be reversed and the relative dielectric constant and surface roughness estimated by measuring the reflection values and employing a theoretical reflection model.
In statistics and signal processing, the MMSE estimator is a function that minimizes the mean square error (MSE), which is usually referred to as the optimal estimator. The MSE represents the degree of match between the predicted value and the true value, as shown in the following equation:
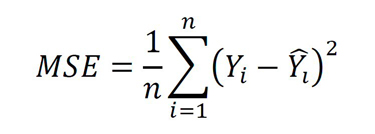
Where 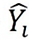 represents the predicted value, Yi is the true value, and n is the sample size.
represents the predicted value, Yi is the true value, and n is the sample size.
When the model has been established but certain parameters are still unknown, these unknown parameters can be estimated by taking the minimum MSE, which is the MMSE method.
The MMSE method is used to set the ranges of εr and hrms, calculate the corresponding theoretical reflection coefficients, compare them with the actual measured values, calculate the MSE, select the theoretical value closest to the measured value and obtain the best values for εr and hrms. Table I shows the εr and hrms values obtained for different materials at different frequencies using the MMSE method.
TABLE I
εr AND hrms VALUES OBTAINED BY THE MMSE METHOD FOR DIFFERENT MATERIALS AT DIFFERENT FREQUENCIES
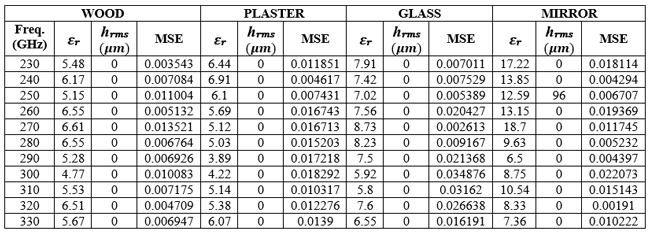
From the estimated values in Table I, the estimated surface roughness values of wood, plaster and organic glass are all zero, which can be understood as these surfaces are considered smooth in this range of wavelengths. However, it cannot be ruled out that the influence of surface roughness is not fully reflected due to insufficient data.
The relationship between relative permittivity obtained by MMSE and frequency is plotted in Figure 8. The estimated values of relative permittivity for three materials; wood, plaster and organic glass are concentrated in a small range, which shows that the relative permittivity is an inherent property of the material, consistent with the measurements.

Figure 8 Relationship between relative permittivity and frequency of four materials obtained using the MMSE method.
The estimation of the relative permittivity and surface roughness for the mirror is not ideal, as shown by the large fluctuation in relative permittivity, and the surface roughness only appears at a specific frequency, which is unusual. It is believed that this anomaly is related to the presence of metals in the mirror’s composition. In fact, a similar measurement conducted with an iron plate yielded unexpected results as well. Not only were estimated values inconsistent with those determined through the MMSE method, but the relationship between the reflection coefficient and the angle or frequency did not match the theory. We believe that the reflective properties of metal surfaces are quite different from those of non-metals and other factors must be considered. This requires further exploration in future experiments.
CONCLUSIONS
The reflection characteristics of common materials at THz frequencies are investigated based on measurements from 220 to 330 GHz of the reflection path and analyzing the relationship between reflection coefficients, incident angles and frequencies. The experimental results show that the reflection coefficient depends on the type of material when a vertically polarized wave is incident.
The reflection characteristics of non-metallic materials behave in accordance with theory, increasing with frequency and incident angle. The reflection properties of metallic materials, however, differ significantly from theory and require further investigation.
The use of the MMSE method for estimating the relative dielectric constant and surface roughness of reflective materials introduces a new approach for studying reflection characteristics.
Future work will focus on measuring different types of reflective materials using finer frequency steps and finer spatial densities based on this system to enrich the reflective channel characterization database. The measurement of the dielectric constant and surface roughness of reflective surfaces using this system will also require a finer and more extensive accumulation of measurements. THz reflection characteristics of metal surfaces with multiple/multipath reflections to explore the transmission performance of THz indoor channels in greater depth will be explored as well.
References
- “6G Overall Vision and Potential Key Technologies,” IMT-2030(6G) Promotion Group, White Paper, June 2021.
- “6G Typical Scenarios and Key Capabilities,” IMT-2030(6G) Promotion Group, White Paper, 2022.
- “The Next Hyper-Connected Experience for All,” Samsung, July 2020.
- H. Tataria, M. Shafi, A. F. Molisch, M. Dohler, H. Sjöland and F. Tufvesson, “6G Wireless Systems: Vision, Requirements, Challenges, Insights and Opportunities,” Proceedings of the IEEE, Vol. 109, No. 7, July 2021, pp. 1166-1199.
- “Research on Channel Measurement and Modeling for 6G,” IMT-2030(6G) Promotion Group, 2022.
- P. H. Siegel, “Terahertz Technology,” IEEE Transactions on Microwave Theory and Techniques, Vol. 50, No. 3, March 2002, pp. 910-928.
- T. Haoyu, T. Pan and Z. Jianhua, “A Review of Research on Terahertz Channel Characteristics and Modeling for 6G,” Mobile Communications, Vol. 44, No. 6, 2020, pp. 29-35.
- X. Sha, Li Haoran, Li Lingxiang, et al. “Review of Terahertz Communication Technology, Journal of Communications, Vol. 41, No. 5, 2020, pp. 168-186.
- K. Sato, H. Kozima, H. Masuzawa, T. Manabe, T. Ihara, Y. Kasashima and K. Yamaki, “Measurements of Reflection Characteristics and Refractive Indices of Interior Construction Materials in Millimeter-Wave Bands,” Proceedings of the IEEE 45th Vehicular Technology Conference. Countdown to the Wireless Twenty-First Century, Vol. 1, July 1995, pp. 449-453.
- R. Piesiewicz, C. Jansen, D. Mittleman, T. Kleine-Ostmann, M. Koch and T. Kurner, “Scattering Analysis for the Modeling of THz Communication Systems,” IEEE Transactions on Antennas and Propagation, Vol. 55, No. 11, November 2007, pp. 3002-3009.
- J. Ma, R. Shrestha, L. Moeller and D. M. Mittleman, “Channel Performance for Indoor and Outdoor Terahertz Wireless Links,” APL Photonics, Vol. 3, No. 5, February 2018.
- P. Tang, J. Lin, J. Zhang, L. Tian, Z. Chang, L. Xia and O. Wang, “Research on Reflection Characteristics of Terahertz Channel for 6G,” Journal of Communications, Vol. 43, No. 5, 2022, pp. 102-109.
- Y. Yang, W. Wang, B. Tang, Y. Wu, J. Jia, H. Li and Y. Liu, “Channel Measurements and Propagation Characterization for Indoor Terahertz Communication,” International Journal of RF and Microwave Computer-Aided Engineering, Vol. 32, No. 10, October 2022.
- M. Wang, W. Li, J. Ding and Y. Wang, “Reflection Characteristics Measurements of Indoor Wireless Link in D-Band,” Sensors, Vol. 22, No. 18, September 2022.
- O. Landron, M. J. Feuerstein and T. S. Rappaport, “In Situ Microwave Reflection Coefficient Measurements for Smooth and Rough Exterior Wall Surfaces,” Proceedings of the IEEE 43rd Vehicular Technology Conference, May 1993, pp. 77-80.
- A. Bose and C. H. Foh, “A Practical Path Loss Model for Indoor WiFi Positioning Enhancement,” Proceedings of the 6th International Conference on Information, Communications & Signal Processing, December 2007.
- O. Landron, M. J. Feuerstein and T. S. Rappaport, “A Comparison of Theoretical and Empirical Reflection Coefficients for Typical Exterior Wall Surfaces in a Mobile Radio Environment,” IEEE Transactions on Antennas and Propagation, Vol. 44, No. 3, March 1996, pp. 341-351.
- T. S. Rappaport, Wireless Communications: Principles and Practice, Vol. 2, Prentice Hall, Hoboken, NJ, USA, 1996.
- P. Beckmann and A. Spizzichino, The Scattering of Electromagnetic Waves from Rough Surfaces, Artech House, Norwood, MA, USA, 1987.

