FABRICATION

Figure 3 Fabricated SAW filters.

All SAW filter variations are fabricated on a wafer from one mask. Figure 3 shows photos of the wafer and the pattern of fabricated filters. A 128-degree Y-cut LiNbO3 wafer is chosen because of its large piezoelectric coupling coefficient. First, the wafer is spin-coated with an AZ 5214E photoresist. Then, the pattern mask is mounted on the spin-coated wafer with a soft contact and exposed to UV light. Polymerization of the photoresist layer by the UV light decreases its solubility so that after washing, only the UV-exposed photoresist is left on the wafer.

The wafer with patterned photoresist is transferred to the sputtering system where 20 nm of silver and 180 nm of copper are uniformly sputtered onto the wafer disregarding the existence of the photoresist layer. The silver layer increases the adhesion of the copper.

Finally, the sputtered wafer is soaked in acetone. The photoresist layer is removed together with the metal layer from its top so that the wafer with the desired pattern remains.

The fabrication process is illustrated in Figure 4.

Figure 4 SAW filter fabrication process.

EXPERIMENTAL RESULTS

Figure 5 shows a filter being measured at the probe station. S-parameters (S₁₁ and S₂₁) are measured with a vector network analyzer.

Figure 5 Probe station measurement.

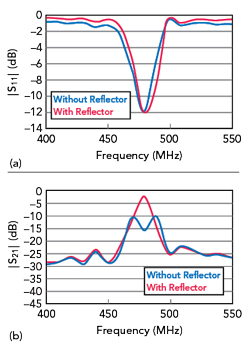
Figure 6 Impact of reflectors on filter performance: measured reflection coefficient |S₁₁| (a) and transmission coefficient |S₂₁| (b) with and without reflectors.

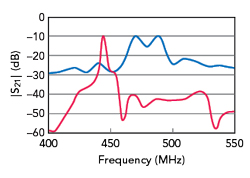
Figure 7 Comparison of measured (blue line) and simulated (red line) |S₂₁| of a saw filter without reflectors.

The impact of the reflectors can be observed in Figure 6, where the filter with reflectors displays significantly better transmission performance. The filter with reflectors has a higher |S₂₁| (about -3 dB) at the resonance peak, while without reflectors it is less than -10 dB around the peak. In Figure 6a, |S₁₁| of both designs is almost identical. Therefore, it can be concluded that the difference in |S₂₁| depends to a greater degree on reception versus transmission. The induced mechanical waves are reflected between the two ports by the reflectors so that the same wave is transmitted to the receive port multiple times. Therefore, the filter with reflectors provides better performance.

Measured and simulated |S₂₁| of the SAW filter without reflectors are shown in Figure 7. The resonant frequency is shifted from 440 MHz in the simulation to 480 MHz in the measurement. The difference is attributed to the simplified 2D modeling approach of the 3D structure. However, it does provide a close prediction of |S₂₁|.

Figure 8 shows the results of the remainder of the parametric study. Figure 8a compares |S₂₁| of filters with different IDT overlapping lengths. A length of 20 μm is insufficient to fully activate electromechanical coupling, while 100 and 200 μm are sufficient. The measurement of lengths between 20 and 80 μm will be needed if a minimal design is desired; however, a 200 μm overlapping length shows only a slight shift in the resonance peak and broadens the bandwidth.

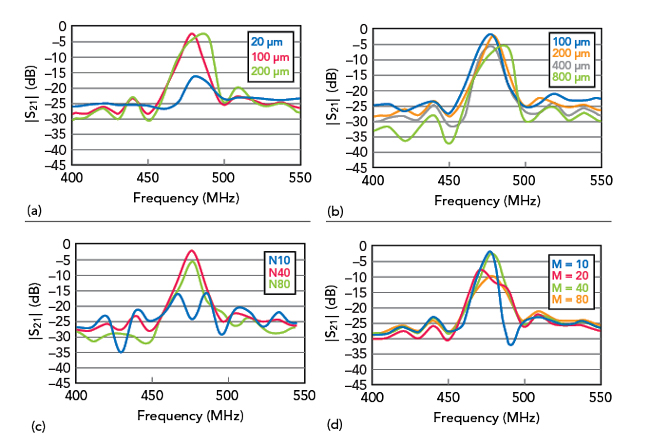
Figure 8 Transmission coefficient |S₂₁| as a function of IDT overlap (a), port separation (b), number of IDTs per port (c) and number of reflectors per port (d).

Figure 8b illustrates the impact of port separation on |S₂₁|. Greater attenuation is expected with increasing separation. The filter with 800 μm in separation has about 3 dB greater attenuation than those with 100 and 200 μm. As the separation is increased to 800 μm, a wider bandwidth and a shift in the resonance peak is observed.

Both the numbers of IDTs (N) and reflectors (M) are varied independently (see Figures 8c and 8d). The number of reflectors per port is fixed to 40 when varying the number of IDTs per port and vice versa. The best performance (|S₂₁| less than -3 dB) is achieved when M = 10 or 40. When M = 80, the peak value of |S₂₁| drops to -10 dB. This implies that fewer reflectors are needed to effectively reflect the incident mechanical waves. An excessive number of reflectors or non-unity ratio between IDTs and reflectors appears to deteriorate performance.

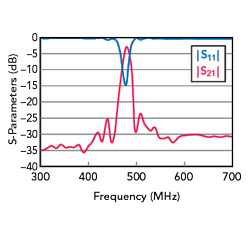
Figure 9 Measured |S₁₁| and |S₂₁| of the optimized filter.

The best performing SAW filter is one with 40 pairs of reflectors and IDTs per port, greater than 100 μm in IDT length and 100 μm or less of port separation. It demonstrates less than 3 dB of insertion loss and greater than 10 dB of return loss at 480 MHz without external matching elements at the ports (see Figure 9).

CONCLUSION

The investigation of the impact of SAW features on performance is demonstrated through an in-house fabrication capability to manipulate detailed features with a short design-fabrication-measurement-redesign cycle. An entire design-fabrication-measurement procedure is established and serves as a foundation to meet future requirements for sensing and telecommunication components.

ACKNOWLEDGMENT

This work was supported by the Internal Research Grant and The Dean’s Research Fund of the Faculty of Liberal Arts and Social Sciences from the Education University of Hong Kong (Project No: R4348 and FLASS 04717) and an IEEE-MTTS undergraduate scholarship. The fabrication facilities were provided by the University Research Facility at The Hong Kong Polytechnic University (PolyU). The measurements were carried out of Dr. Mung’s laboratory.

References

1. S. Moench, J. M. Meyer, A. Žukauskaite, V. Lebedev, D. Fichtner, J. Su, F. Niekiel, T. Giese, L. Thormählen, E. Quandt and F. Lofink, “AlScN-Based SAW Magnetic Field Sensor for Isolated Closed-Loop Hysteretic Current Control of Switched-Mode Power Converters,” IEEE Sensors Letters, Vol. 6, No. 10, October 2022.
2. J. Labrenz, A. Bahr, P. Durdaut, M. Höft, A. Kittmann, V. Schell and E. Quandt, “Frequency Response of SAW Delay Line Magnetic Field/Current Sensor,” IEEE Sensors Letters, Vol. 3, No. 10, October 2019.
3. S. W. Y. Mung and W. S. Chan, “The Challenge of Active Circulators: Design and Optimization in Future Wireless Communication,” IEEE Microwave Magazine, Vol. 20, No. 7, July 2019, pp. 55–66.
4. S. W. Y. Mung and W. S. Chan, “Tunable Active Three-Way Circulator Using Tunable Transistor Feedback Network,” Electronics Letters, Vol. 53, No. 24, November 2017, pp. 1591–1592.
5. K. Hashimoto, Surface Acoustic Wave Devices in Telecommunications, Springer, Berlin/Heidelberg, Germany, 2000.
6. R. Aigner, “SAW and BAW Technologies for RF Filter Applications: A Review of the Relative Strengths and Weaknesses,” Proceedings of the IEEE Ultrasonics Symposium, November 2008, pp. 582–589.
7. D. Morgan, Surface Acoustic Wave Filters with Applications to Electronic Communications and Signal Processing, Elsevier, U.K., 2007.
8. K. Hashimoto, G. Endoh and M. Yamaguchi, “Coupling-of-Modes Modelling for Fast and Precise Simulation of Leaky Surface Acoustic Wave Devices,” Proceedings of the IEEE Ultrasonics Symposium, Vol. 1, November 1995, pp. 251–256.
9. J. H. Kuypers and A.P. Pisano, “Green’s Function Analysis of Lamb Wave Resonators,” Proceedings of the IEEE Ultrasonics Symposium, November 2008, pp. 1548–1551.
10. T. Wang, R. Green, R. Guldiken, J. Wang, S. Mohapatra and S.S. Mohapatra, “Finite Element Analysis for Surface Acoustic Wave Device Characteristic Properties and Sensitivity,” Sensors, Vol. 19, No. 8, April 2019.
11. C. Campbell and J. C. Burgess, “Surface Acoustic Wave Devices and Their Signal Processing Applications,” Journal of the Acoustical Society of America, Vol. 89, March 1991, pp. 1479–1480.
12. A. K. Namdeo and H. B. Nemade, “Simulation on Effects of Electrical Loading Due to Interdigital Transducers in Surface Acoustic Wave Resonator,” Procedia Engineering, Vol. 64, 2013, pp. 322–330.