Evaluating the realistic performance of antennas integrated into complex setups has been a long-term challenge, currently relevant to automotive connectivity, for example. Numerical electromagnetic (EM) modeling offers a cost-effective alternative to device testing but is often limited by an incomplete or inaccurate knowledge of the device under test (DUT). While this shortcoming does not apply to test approaches, the implementation of adequate measurement systems, such as full-vehicle over-the-air (OTA) test setups, requires large investments and, possibly, a significant amount of space. We present a way to converge both worlds in an optimal cost-benefit technique by combining antenna measurements with full-wave simulation.
Three-dimensional EM modeling tools have seen extraordinary technical and commercial development since the early 90s. Now, there is almost no problem involving Maxwell’s equations which cannot be solved with the right software and computational resources. Nevertheless, the need for RF measurements continues to grow, and simulations are not foreseen as a substitute for testing soon. The main reason is that full-wave simulation, even performed by an expert, is only as good as the knowledge of the problem modeled. In most circumstances, this knowledge is incomplete, even for the device manufacturer. Taking the example of a mobile phone, some components come from suppliers, material dielectric properties are not fully characterized, manufacturing tolerances and operating uncertainties of the components are not completely accounted for, etc.
Measurements do not suffer from a similar lack of knowledge, as no simplifying assumptions are required about the DUT. However, the complexities of experimental setups, including realistic integration or usage conditions, must be considered. For example, for autonomous driving cars using cellular vehicle-to-everything (C-V2X) technology for data communication, the connectivity in cars is developing to unprecedented levels. Evaluating the accurate performance of all transceivers (i.e., cellular, WLAN, GNSS, etc.) necessitates including the impact of the complete car on all radiated emissions. To address these aspects, the 5GAA organization is currently standardizing the use of full-vehicle OTA testing systems using 10 m or larger anechoic chambers (see Figure 1).1 The corresponding costs are as substantial as the space requirements, and the realizable test scenarios are still limited. A close to real-world test solution would require unreasonable effort, time and cost for measuring the OTA performance of an integrated 5G mmWave antenna module, and no test chamber can assess the effects of various grounds on the measured metrics or an actual link budget in a car-to-car transmission.
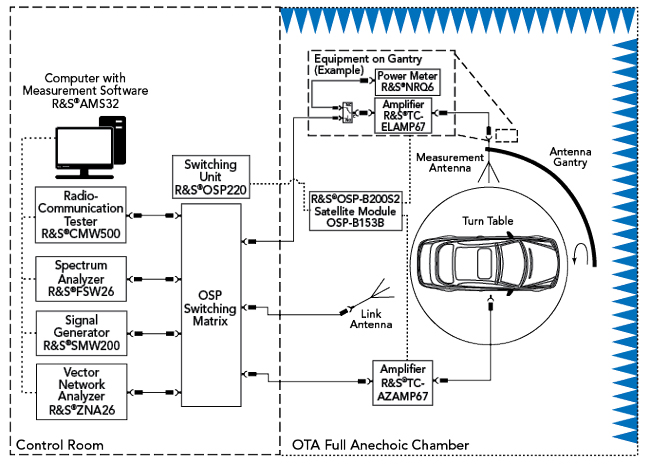
Figure 1. R&S full-vehicle OTA testing system based on draft 5GAA requirements.
To overcome the limitations in both antenna simulation and measurement, the best of both numerical and experimental methods can be combined: measure the part of the problem which cannot be accurately modeled and compute the portion which is too costly or complex to measure.
MEASUREMENT + SIMULATION

Figure 2. Equivalent source calculated from complex voltage measurements over a sphere.

Figure 3 Steps in the measurement-simulation technique.
Combining measurement and simulation is a straightforward outcome of the fundamentals of wave physics. From the Huygens-Fresnel principle or the equivalence theorem, the six components of the electric (E) and magnetic (H) field vectors outside of a volume, Vs, encompassing a radiating source within a surface, Σs, can be exactly computed from only two of the EM field components over Σs or the associated surface electric and magnetic currents J and M (see Figure 2). Conversely, proven techniques can solve the inverse problem: calculating the currents J and M from two characterized components of E and/or H over a closed surface, Σm, containing Σs.2-5
Imagine that Σm is a sphere surrounding an antenna. A test probe scans Σm and delivers two voltages at each sampling point, which relate to the local, orthogonal, spherical coordinate phasor components Eθ and Eφ. Knowing the magnitude and phase of these voltages over the sphere is then sufficient to derive a model of the source, equivalent to the antenna under test, in the sense that the model generates the same E and H fields outside of Σs. This principle is not limited to a spherical surface; a sphere matches the results where the measurements are obtained using a spherical test range.
