Technical Feature
Intermodulation Products for a Mixer Subjected to a Multi-carrier Signal
Applying a multi-carrier signal to a mixer leads to a large range of intermodulation products. To perform a link budget of a multi-carrier transmit-receive system, it is important to predict the location and magnitude of those intermodulation products. To achieve this, several methods, including Volterra series and harmonic balance, have been used. The harmonic balance method has been incorporated in microwave circuit simulators, but fails to give accurate results when dealing with a large number of carriers. This article shows how a transient simulation can be set-up to predict the behavior of the intermodulation products in a multi-carrier environment using a mixer nonlinear model.
Assaad Borjak and Taoufik Bourdi
Resonext Communications Inc.
San Jose, CA
New modulation schemes in cellular systems have recently been proposed in which multi-carrier signals are a must. In transmission systems with multi-carrier signals, it is paramount to predict the behavior of the mixer and the location and amplitude of the intermodulation products generated by the mixer. The intermodulation products generated by the mixing of a two-tone signal with a local oscillator are well known and can easily be predicted mathematically and via simulation. Simulation of a two-tone signal mixing with a local oscillator is usually performed using the harmonic balance simulation in a microwave circuit simulator. However, with an increase in the number of carriers, it becomes extremely difficult to use harmonic balance simulation to predict the behavior of the intermodulation products. This is because the harmonic balance fails to converge, giving erroneous results.
The treatment of the intermodulation products in a multi-carrier environment has recently received a great deal of attention in the literature. Pedro, et al.1 have derived mathematical equations for parameters describing the nonlinearity in a multi-carrier set-up. The aim of this article is to introduce a method for predicting the behavior of a mixer subjected to a multi-carrier signal and to show how to simulate such an arrangement in a microwave circuit simulator.
Basic Description of Intermodulation in Mixers Subjected to a Multi-carrier Signal
A mixer is a very nonlinear device. To characterize it, several people have proposed nonlinear models. Before these models are described, the frequency spectrum of a mixer (assumed to be linear multiplier) is shown first and then the nonlinear device model will be developed progressively and used to simulate and predict a mixer undergoing a multi-carrier signal.

Fig. 1 The spectrum of the mixer output in a transmitter for three cases;
mixer is a linear multiplier with the multi-carrier band starting at (a) f = 0 and (b) f = fB1
and (c) mixer is a nonlinear device with the multi-carrier band starting at f = fB1 .
Figure 1 illustrates the spectrum of an upconverter mixer output for three different cases. In the first case, considering the linear model of a mixer as a multiplier, the relationship becomes

For simplicity, if all the carriers are of equal amplitude and have a phase of 0°, and the amplitude is normalized to 1, Equation 1 becomes

Then, the results of the mixing yield intermodulation frequencies at fLO + fi and fLO - fi
where
i = 1, 2, … N
This is illustrated for a multi-carrier signal with the first frequency at f = 0. As a rule of thumb, to obtain the intermodulation products the multi-carrier band is translated to the upper-side of the local oscillator frequency to yield the positive intermodulation products fLO + fi and then the results are mirrored around the fLO axis to obtain the negative intermodulation products fLO - fi . In the second case, the same procedure is applied to a multi-carrier signal but with a first frequency offset from f = 0. However, in real life, the mixer is a nonlinear element and would never behave like a multiplier. The nonlinearity can be described by the polynominal equation

Of course, this equation gives the intermodulation products at frequencies of the form ±mLO fLO ±m1 f1 ±m2 f2 ±m3 f3 ±m4 f4 ±… ±mN fN . The products that are located around the frequency of transmission are of interest. Therefore, the intermodulation products around the local oscillator frequency are
fIMD = fLO ±m1 f1 ±m2 f2 ±m3 f3 ±m4 f4 ±… ±mN fN (4)
As can be seen from this equation, the intermodulation products are surrounding the local oscillator frequency fLO. Hence, the same reasoning can be used as in the multiplier case. First the intermodulation products of the multi-carrier signal are studied and described by
±m1 f1 ±m2 f2 ±m3 f3 ±m4 f4 ±… ±mN fN (5)
These are located near the low pass area. Then they are translated to the upper-side around the local oscillator frequency and mirrored around the oscillator frequency fLO to get the lower side of the intermodulation products. This is the third case illustrated.
Equation 5 shows that there are several orders of intermodulation products: first, second-order products, then third-order, fourth-order, fifth-order products, etc. Second-order products are of two categories: second-order sum products of the form fi + fj and 2fi and second-order subtract products of the form fi - fj . Third-order products also have two categories: third-order sum products of the form 3fi , 2fi + fj and fi + fj + fk and third-order subtract products of the form 2fi - fj and composite triple beat fi + fj - fk , fi - fj + fk , -fi fj + fk . Kos, et al.2 derived the number of these beats. It should be mentioned here, that higher order products might fall on lower order products but hopefully higher order products have small amplitudes. For example, fifth-order products might fall on third-order products, etc.
Intermodulation Simulation of a Mixer
The objective here is to outline a method to simulate mixer intermodulation products in a microwave circuit simulator such as Agilent-ADS and to show how it can produce results like those described previously. A transient simulation is used since harmonic balance failed to give accurate results, especially for a large number of carriers (24, for example).
The mixer nonlinear model used in this article is a mathematics-based device model similar to that suggested by Parker and Skellern.3 It was implemented using the symbolic-defined device function in ADS. As an example, the behavior of an IQ mixer used in a transmitter as an upconversion stage is predicted. The IQ mixer is being subjected to a 24-carrier signal of 14 MHz minimum frequency and 600 kHz carrier spacing. The local oscillator frequency used is 900 MHz. This is illustrated in Figure 2 . In this case, open-circuit transmission lines are used to block the local oscillator frequency from the 24-carrier source.

Fig. 2 Transmitter nonlinear IQ mixer simulation set-up.
While setting-up the simulation, settling time, frequency resolution, numerical noise floor and windowing must be defined. For accurate fast fourier transform (FFT) of the time-domain data, only steady-state data must be used. Hence, an extra 0.2 ms simulation time is added to take care of the initial transient (non-steady-state) simulation results. Then, the initial 0.2 ms section of data is dumped when performing time-to-frequency conversion. As for the frequency resolution, the frequencies chosen are multiples of the lowest frequency. In this case, the lowest possible frequency is 200 kHz. The frequency resolution is directly related to the simulation stop time.
To obtain a very low noise floor, a small maximum simulation time step is chosen. While performing FFT on time-domain data, a Hanning window was selected since it offered the lowest noise floor. It should be noted that no windowing is necessary if it is ensured that each frequency is integrated over an integer number of periods.
Figure 3 shows the results of the IQ mixer upconverter frequency spectrum. All second- and third-order sum and subtract intermodulation products can be seen as predicted. Some higher order components could also be seen buried under those intermodulation products.

Fig. 3 Simulation results for the upconverter IQ mixer showing the
intermodulation products of different orders with upper and lower side 24 carriers;
(a) mixing carriers in low pass area and (b) upconverted carriers.
The same procedure can be done to check the behavior of a mixer in a receiver. Figure 4 shows the basic behavior of a downconverter mixer, subjected to a multi-carrier signal. In part A of this figure, the mixer is considered as a linear multiplier. Hence it has two downconverted sets of frequencies. The first downconverted set is translated to low pass and the second is an image of the low pass set, and can be treated as a mirror of the multi-carrier at f = fLO with different amplitude. The same interpretation can also be applied to the linear mixer of part B. When the mixer is considered a nonlinear device, the frequency spectrum is derived by generating the intermodulation products of the multi-carrier signal at f = fLO + fB1 and then translated down to the low pass area to get the first downconverted signal. The second downconverted signal is an image of the low pass. Simulation set-up for this downconverted case is similar to that of the upconverted case shown previously and will not be discussed here for brevity.
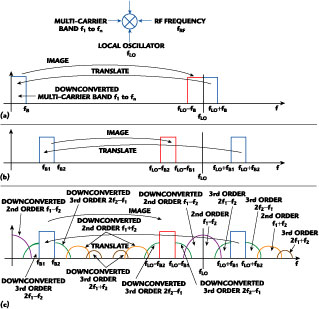
Fig. 4 The spectrum of the mixer output in a receiver for three cases;
mixer is a linear multiplier with the multi-carrier band starting at (a) f = fLO and
(b) f = fLO + fB1 , and (c) mixer is a nonlinear device with the multi-carrier
band starting at f = fLO + fB1 .
Conclusion
A simulation set-up to predict the intermodulation products of a nonlinear mixer used as an upconverter in a transmitter and a downconverter in a receiver is presented. The simulation is very useful in predicting location and magnitude of intermodulation products and can easily be used in a microwave circuit simulator.
References
1. J.C. Pedro and N. Carvalho, "On the Use of Multi-tone Techniques for Assessing RF Components' Intermodulation Distortion," IEEE Transactions on Microwave Theory and Techniques , Vol. 47, No. 12, December 1999, pp. 2393-2402.
2. T. Kos, B. Zovko-Cihlar and S. Grgic, "An Algorithm for Counting of Intermodulation Products in Multi-carrier Broadband Systems," IEEE ISIE 1999 , Bled, Slovenia, pp. 95-98.
3. A.E. Parker and D.J. Skellern, "A Realistic Large-signal MESFET Model for SPICE," IEEE Transactions on Microwave Theory and Techniques , Vol. 45, No. 9, September 1997, pp. 1563-1571.
4. M. Leffel, "Intermodulation Distortion in a Multi-signal Environment," RF Design , June 1995, pp. 78-84.
5. N. Carvalho and J. Pedro, "Two-tone IMD Asymmetry in Microwave Power Amplifiers," IEEE MTT-S Symposium Digest , 2000.
Assaad Borjak is a principal design engineer at Resonext Communications Inc., San Jose, CA. He was awarded a PhD degree in electrical engineering in 1993 from the Institute of Science & Technology at the University of Manchester (UK). Dr. Borjak's current work is in the area of RF CMOS mixed signal IC design for wireless transmission systems. This includes current-steering DAC, pipeline ADC architectures and delta-sigma fractional-N synthesizers. His previous tenure was at Nokia Networks (UK), where he was an RF specialist. His main interests are RFIC and mixed signal IC circuits, systems and architectures for wireless transmission sytems. Dr. Borjak is a senior member of IEEE and can be reached via e-mail at assaad.borjak@resonext.com.
Taoufik Bourdi is a senior member of the technical staff at Resonext Communications Inc., Irvine, CA. His current work is in the area of mixed signal/RFIC CMOS design for wireless transmission systems. This includes the design and implementation of oversampled DAC/ADC and Delta Sigma fractional-N frequency synthesizer architectures. Previously, at Nokia Networks (UK), where he was a senior design engineer, his work involved the development of new RF technology architectures including fast frequency hopping synthesizers for the GSM/DCS and PCS transceivers. Mr. Bourdi is also a PhD candidate in mixed signal IC at Westminster University (UK). His main interests are circuits, systems and novel RF architectures for wireless communication systems. Mr. Bourdi can be reached via e-mail at taoufik.bourdi@resonext.com.
