A Large Bandwidth T-shaped Microstrip-fed Ground Plane Slot Antenna

Technical Feature

A Large Bandwidth T-shaped Microstrip-fed Ground Plane Slot Antenna

The characteristics of a T-shaped microstrip feeding a ground plane slot antenna have been analyzed using the finite difference time domain (FDTD) method. The impedance and bandwidth of the proposed antenna depend highly on the design parameters. The radiation resistance has a low value. The measured bandwidth is approximately 39.6 percent for |S₁₁ | ≥ 10 dB.

Yong-Woong Jang
College of Keukdong
Eumsung, Korea

Jeong-Chull Yoon
ACE Technology Co.
Chatsworth, CA

Ho-Sub Shin
National University of Chungbuk
Chungbuk, Korea

Microstrip antennas offer the advantages of thin profile, light weight, low cost, conformability to a shaped surface and compatibility with integrated circuitry. The slot antenna has been investigated since the 1940s,¹ and is treated in many electromagnetic text books.^2,3 The major drawback of a microstrip antenna, in its basic form, is its inherently narrow bandwidth. This is a major obstacle to its wide application.

In a conventional microstripline-fed slot antenna, a narrow rectangular slot is cut in the ground plane, and the slot is excited by a microstrip feedline with either a short⁴ or an open⁵ termination. With this feed configuration, a good impedance match has been achieved for a narrow slot, and an impedance bandwidth of approximately 20 percent has been obtained.⁶ However, as the width of the slot increases, the radiation resistance of the slot antenna increases proportionately. This, in turn, reduces the impedance bandwidth of the antenna even though the size of the slot is larger.⁷ Shum, et al.⁸ have shown the possibility of increasing the bandwidth of the wide slot antenna by terminating the open end of the feedline within the width of the slot; however, substantial bandwidth improvement has not been achieved with this approach.

In this article, the impedance of the T-shaped microstrip feedline structure and the conventional feeding structure have been analyzed using the FDTD method. Good impedance match is obtained with a conventional feeding structure, only for narrow slots. However, a T-shaped feedline is able to match the input impedance for a narrow as well as a wide slot antenna. When the T-shaped feedline is used, the bandwidth is extended proportionately to the slot width and the radiation resistance has a low value. The characteristics of a printed slot antenna fed with a T-shaped microstrip line have been studied. A bandwidth wider than for the conventional feed has been obtained. From these results, an antenna having a broad bandwidth was designed, fabricated and measured.

FDTD Formulation

The FDTD method is formulated by discretizing Maxwell's curl equations over a finite volume and approximating the derivatives with central difference approximations. These finite difference time domain approximate equations contain a second-order error in both space and time steps. According to Yee's notation,⁹ the space point in the FDTS cell is (iD x, jDy, kDz), the time increment is nDt and the arbitrary function is represented as F(iD x, jDy, kDz, nDt). In the analysis of the microstrip slot antenna design, the Mur's absorbing boundary condition¹⁰ was applied. The time domain value, which is calculated by a finite time domain method,¹¹ is Fourier-transformed and the response value in the frequency domain can be calculated. Since the microstrip feedline is an open stub, the microstrip antenna is a one-port circuit. Therefore, the reflection coefficient S₁₁ of the microstrip antenna is given by

where

V₁ ^ref(t) = reflected voltage
V₁ ^inc(t) = incident voltage
F = Fourier transform notation

From the calculated reflection coefficient, the voltage standing wave ration (VSWR) can be obtained from

The percentage bandwidth of the antenna was determined from the impedance data. From now on the term bandwidth will refer to percentage bandwidth unless otherwise specified. The bandwidth is defined as

where

f_r = resonance frequency
f_r1 and f_r2 = frequencies for which the magnitude of the reflection coefficient is less than or equal to 1/3 (corresponding to a VSWR ≤ 2)

The electric field of the far-field patterns can be calculated as

where

k = propagation constant
E_m = electric field at the slot
W = slot width
L = slot length

Antenna Structure and Simulation Results

The structure of the proposed antenna, as shown in Figure 1 , consists of the slot radiator and a T-shaped feedline. A T-shaped microstrip feedline is proposed to match the input impedance for narrow as well as wide slot antennas. When a T-shaped feedline is used, the bandwidth can be broadened proportionately to the slot width with good impedance matching. This type of antenna is better than the conventional feedline structure. The substrate used has a dielectric constant of 4.3 and a thickness of 1.0 mm. The slot is l_s and its width is W_s . The length of the horizontal branch of the T is l_d . The offset is the distance between the slot center and the center of the microstrip line; W_f is the width of the feedline. To analyze the antenna correctly, Dy and Dx are chosen so that an integral number of nodes fit the feedline and slot exactly. Dz is chosen so that an integral number of nodes fit the thickness h of the substrate exactly. The sizes of the space cells are Dx = 0.3214 mm, Dy = 0.25 mm, Dz = 0.333 mm. The total analysis space is composed of 280 x 360 x 43 cells in the x, y, z directions, respectively. The size of the antenna elements used in the analysis are l_s = 156 Dx, W_s = 64 Dy, l_d = 110 Dx, offset = 18 Dy, W_f = 6 Dx. In order to calculate the input S-parameters, a standard technique of time gating the signal on the microstrip line is used to separate the incident and reflected waveforms. The S-parameters are obtained from the ratio of the Fourier transforms of these waveforms. The simulation is allowed to continue until the energy reflected from the resonant cavity toward the source has been reduced to a negligible level. Stopping the run too early results in ripples on the calculated S-parameters.

Figure 2 shows the three-dimensional E_z -component of the electric field as the time-varying pulse has reached a steady state. The time step is 1.9 ps to satisfy the Courant stability condition.¹² The pulse width is 32 time steps. The Gaussian pulse is excited just underneath the dielectric interface of the antenna.

Figure 3 shows the comparison of the normalized (to 50 W) radiation resistance of a slot antenna with the proposed and the conventional feeding structures. The conventional center-fed transverse slot antenna has a large value of radiation impedance. This case is good for the impedance matching of a narrow slot antenna only. But a T-shaped feedline is able to match the input impedance of not only a narrow but also a wide slot antenna. When the T-shaped feedline is used, the radiation resistance does not change from its low value (the normalized resistance value is approximately 1.0) as a function of the slot width.

The characteristics of the antenna are sensitive to the design parameters (l_s , W_s , l_d , offset, W_f ). The input impedance, return loss and radiation pattern in the frequency domain are calculated by taking the Fourier transform of the time domain results.

With the other parameters fixed, when the width of the slot is varied from 8 to 32 mm with a constant cell size Dy = 0.25 mm, the normalized impedance results are shown in Figure 4 . When W_s is 16 mm, the normalized radiation resistance and reactance are approximately 1.0 and zero, respectively, at the center frequency of 2.3 GHz. Good impedance matching is obtained for W_s = 16 mm at the center frequency of 2.3 GHz.

With the other design parameters fixed, when the offset position is varied from 0 to 5.5 mm, the normalized impedance results are shown in Figure 5 . When the offset is 0 mm, the normalized radiation resistance is approximately 3.5 at 2.3 GHz. However, when the offset is 4.5 mm, the normalized radiation resistance and reactance are about 1.0 and 0, respectively, at the center frequency. This means that the antenna is resonant at the center frequency of 2.3 GHz for an offset = 4.5 mm.

Table 1 shows the optimized design parameters of the proposed structures and their bandwidth as a function of the slot width.

Experimental Results

The proposed antenna was fabricated using an FR-4 substrate (e_r = 4.3, h = 1.0 mm) and the ground plane size of the two-element microstrip slot antenna array is 230 mm x 120 mm. The measurements were performed with an HP8510B network analyzer.

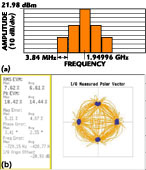
Figure 6 shows the measured impedance locus. The input impedance of the antenna exhibits a broad bandwidth characteristic, which is to be contrasted with the narrow band characteristic of the conventional microstripline-fed structure. The measured results are in good agreement with the calculated results.

The calculated and measured return loss of this antenna are compared in Figure 7 . The measured results are in good agreement with the FDTD results. The measured bandwidth of the antenna is 1.8 to 2.71 GHz, which is approximately 39.6 percent for |S₁₁ | ≥ 10 dB, at the center frequency of 2.3 GHz. The measured bandwidth (39.6 percent) is wider than the simulation result (39.1 percent).

Figure 8 shows the experimental radiation pattern in the x-z plane at f = 2.3 GHz. After calibration using a horn antenna, the far field radiation pattern was measured. The beamwidth is approximately 70°.

Conclusion

The characteristics of a T-shaped microstripline-fed printed slot antenna are presented. It was found that the bandwidth of the antenna depends highly on the design parameters. The proposed antenna has low radiation resistance and wide band characteristics. The experimental bandwidth is approximately 39.6 percent. This antenna may be useful in broadband antenna arrays.

References
1. H.G. Booker, "Slot Aerials and Their Relation to Complementary Wire Aerials," J. IEE (London) , Part IIIA, Vol. 93, 1946, pp. 620-626.
2. Robert E. Collin, Antenna and Radiowave Propagation , McGraw-Hill, NY, 1985.
3. R.Garg, P. Bhartia, I. Bahl and A. Ittipiboon, Microstrip Design Handbook , Artech House, MA, 2001, Chapter 7.
4. Y. Yoshimura, "A Microstrip Slot Antenna," IEEE Transactions on Microwave Theory and Techniques , Vol. 20, No. 11, November 1972, pp. 760-762.
5. D.M. Pozar, "Reciprocity Method of Analysis for Printed Slot and Slot-coupled Microstrip Antennas," IEEE Transactions on Antennas and Propagation , Vol. AP-34, December 1986, pp. 1439-1446.
6. A. Axelrod, M. Kisliuk and J. Maoz, "Broadband Microstrip-fed Slot Radiator," Microwave Journal , Vol. 32, No. 6, June 1989, pp. 81-92.
7. M. Kahrizi, T.K. Sarkar and Z.H.Maricevic, "Analysis of a Wide Radiating Slot in the Ground Plane of a Microstrip Line," IEEE Transactions on Microwave Theory and Techniques , Vol. 41, No. 1, January 1993, pp. 29-37.
8. S.M. Shum, K.F. Tong, X. Zhang and K.M. Luk, "FDTD Modeling of Microstripline-fed Wide Slot Antenna," Microwave Optical Technology Letters , Vol. 10, No. 10, October 1995, pp. 118-120.
9. K.S. Yee, "Numerical Solution of Initial Boundary-value Problems Involving Maxwell's Equations in Isotropic Media," IEEE Transactions on Antennas and Propagation , May 1966, Vol. 14, pp. 302-307.
10. G. Mur, "Absorbing Boundary Conditions for the Finite-difference Approximation of the Time-domain Electromagnetic-field Equation," IEEE Transactions on Electromagnetic Compatibility , Vol. 23, No. 4, November 1981, pp. 377-382.
11. D.M. Sheen, S.M. Ali, M.D. Abouzahra and J.A. Kong, "Application of Three-dimensional Finite-difference Time-domain Method to the Analysis of Planar Microstrip Circuits," IEEE Transactions on Microwave Theory and Techniques , Vol. 38, No. 7, July 1990, pp. 849-857.
12. A. Taflove and M.E. Brodwin, "Numerical Solution of Steady-state Electromagnetic Scattering Problems Using the Time-dependent Maxwell's Equations," IEEE Transactions on Microwave Theory and Techniques , Vol. 23, No. 8, August 1975, pp. 623-630.

Yong-Woong Jang received his BS and MS degrees from Myongji University, Seoul, South Korea, in 1989 and 1991, respectively, and his PhD degree from Ajou University, Suwon, South Korea, in 1999. He then became a member of the faculty in the department of electronics communication engineering at the College of Keukdong at Eumsung in Korea. He is now an associate professor. His main areas of interest are antennas, RF-systems and numerical methods in solving electromagnetic problems.

Jeong-Chull Yoon received his BS and MS degrees from Ajou University, Suwon, South Korea, in 1996 and 1998, respectively. From 1998 to 2000 he worked at ACE Technology Co. as a research member. Since 2001 he has been researching at Sam Sung Electro-mechanic joint stock company. His main areas of interest are antennas, RF-systems and EMI/EMC.

Ho-Sub Shin received his BS and MS degrees in computer and communication engineering from the National University of Chungbuk, South Korea, in 1995 and 1998, respectively. He is currently working toward his PhD degree in computer and communication engineering. His research interest is in the area of numerical analysis and modeling of electromagnetic fields and antenna using FDTD.