RF Interference Analysis for Collocated Systems
The proliferation of wireless services has increased the need to collocate systems because of the difficulty in site acquisition due to zoning restrictions, cost and design considerations. However, collocation requires that the resulting RF interference issues be addressed or system performance degradation is risked. This article examines these issues and the measures employed to combat the interference. An analysis procedure and example are also described to determine the collocation parameters that ensure the RF interference is within acceptable limits.
Shailender Timiri
AT&T Wireless Services
Bellevue, WA
RF interference is not unique to collocated systems. However, collocation complicates the interference analysis and control. The interference is dependent on the interactions between the systems, which, in turn, are dependent on the system equipment, system design parameters and site antenna configurations. This scenario can vary widely between systems and locations. For a single system installation, the interference analysis and control are comparatively manageable because of the possibility of restricting site equipment and antennas to a few well-defined configurations. However, for multiple collocated systems it is difficult to predict the scenarios and, other than providing broad guidelines for collocation, there is no option but to perform the interference studies on a site-by-site basis. As the number of collocated sites increases, there will be a growing need to understand and analyze all the issues in a unified manner. This article provides a consolidated study and analysis procedure for RF interference at all sites, collocated or otherwise.
Specifically, this study deals with RF interference issues at a site from the point of view of interference seen at a collocated receiver. Issues such as antenna blockage or antenna field pattern alteration due to collocation are not covered. Typical transmit and receive signal paths and elements are discussed, as well as interference mechanisms and sources, and interference-rejection measures. The analysis procedure is also described, followed by an example.
Transmit and Receive 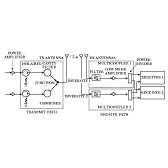
Signal Paths
Figure 1 shows the typical wireless systemís transmit and receive signal paths and their elements. Depending on the system architecture and requirements, signal paths and the elements can vary substantially. In the transmit path, the cavity combiner could be replaced with a hybrid combiner while the use of a linear amplifier or single-channel operation obviates the need for a combiner altogether. Similarly, in the receive path, single-channel operation will not require a multicoupler.
Interference Mechanisms
The discussion on collocation interference mechanisms and their sources assumes all equipment is operating within its published specifications. Therefore, the study of effects such as equipment quality, design parameters (such as automatic gain control or impedance matching) and maintenance process (corroded joints or SWR tuning) is not included. 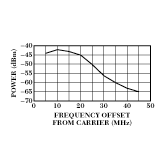
Ideally, the power output from a transmitter should lie completely within the assigned frequency band without any splatter into the adjoining frequencies. However, in reality the output power is spread over a much larger band than the assigned bandwidth, as shown in Figure 2. This out-of-band power is referred to as the broadband, transmitter or far-out noise. Filters placed at the output of the transmitter serve to attenuate the broadband noise but cannot eliminate it completely. The portion of the residual broadband noise that lies in the receiverís in band will enter the receiver and raise its noise floor, effectively reducing the receiver sensitivity. This noise cannot be filtered at the receiver since it occupies the same frequency band as the receiverís desired signals.
Frequently, receiver desensitization is referred to as receiver overload or receiver overdrive. This phenomenon occurs when the receiver is subject to a strong out-of-band signal that can raise the receiver noise floor. Typically, receivers have a wideband frequency response and, therefore, any signal energy (even if it is outside the receiverís desired frequency band) impinging on the receiver can desensitize it. These signals, located within the transmit band of their transmitters, are not filtered at the transmitter. However, since these signals originate from a collocated transmitter, they are out-of-band signals at the receiver and can be filtered out at the receiver input. Filtering attenuates the signal but does not suppress it completely; the residual energy can still adversely affect the receiverís sensitivity.
Intermodulation distortion occurs when signals present in a nonlinear device combine to create new signals as shown in Figure 3.1,2 The device is fed with two sinusoidal input signals and the output is given by the deviceís transfer characteristic, expressed as
vo(t) = 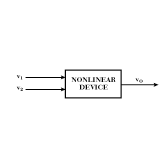
a0 + a1 vi(t) + a2 vi2(t) + a3 vi3(t) + …
(1)
where
vo = output signal
vi = input signal
a0, a1, a2, a3 = constants
vi(t) = v1 + v2
= A1 cos(2pf1t) + A2 cos(2pf2t)
(2)
The first two terms of the transfer characteristic represent the linear response while the third and higher terms represent the nonlinear response of the device. These terms contain the distortion products and are referred to as the nth-order products. For example, the second-order products of the square-law term a2vi2 consist of a DC term, second harmonics of the input frequencies and intermodulation (IM) products, and are described as
Similarly, the cube-law term gives rise to the third-order products, including third-order IM products. The third-order IM products are the most severe in terms of interference and, therefore, the most analyzed. The relationship of the fundamental and third-order IM products is shown in
Figure 4. Table 1 lists the second- and third-order IM products. 

The amplitude of the IM products is a function of the amplitudes of the fundamental (or component) signal levels. Assuming the fundamental signal magnitudes are equal, it can be shown that for each 1 dB change in the fundamental signal level, the third-order IM level will change by 3 dB. This relationship is best seen using an intercept diagram and the concept of the third-order intercept point (IP3), as shown in Figure 5. The diagram consists of plots of the fundamental and third-order IM signal levels plotted against the output or input levels of the fundamental signal. All signal levels are specified using the log scale.
The slope of the third-order IM signal level is three-times that of the fundamental signal level. Intermodulation rejection is the difference between the fundamental and IM signal levels. The IP3, obtained by extrapolation of both lines, is the point at which the IM level is equal to the fundamental level and is a measure of the deviceís nonlinearity. Actual device operation does not extend to the intercept point but is limited to the small-signal region below the 1 dB compression point and well below the intercept point. Therefore, given IP3 and the fundamental signal level, it is possible to determine the corresponding IM level using
IP3o = IP3i + G (4)
where
IP3o = third-order output intercept point in dBm
IP3i = third-order input intercept point in dBm
G = gain in dB
IMR3 = 2 (IP3o ñ Po) (5)
where
IMR3 = third-order intermodulation rejection in dBm
Po = fundamental signal output level in dBm
IM3 = Po ñ IMR3 (6)
= 3Po ñ 2IP3o
where
IM3 = third-order intermodulation signal level in dBm
A more detailed treatment of IM exists,3 but for the purposes of this discussion it will suffice to say that IM signals are generated because of mixing, a nonlinear operation, of two or more signals. The multiple transmit signals at a site can leak into each otherís paths and mix in the base station hardware. The frequencies and power levels of the IM products are a function of the mixing signals and the hardwareís transfer characteristics. IM generation can occur at any of several points along the transmit and receive signal paths but, keeping in mind the scope of this study, the primary sources of IM are the transmit amplifiers, receiver front ends and antennas. IM frequencies that fall within a collocated receiverís in band can affect the receiverís sensitivity severely. Reducing IM interference requires controlling the level of the mixing signals and their frequencies if possible.
Interference Rejection Measures
Since multiple mechanisms and sources of interference exist, it must be emphasized that a combination of interference rejection measures is required to combat the interference. Provided the option exists, frequency planning can be a powerful tool for controlling IM interference. Unlike other measures, frequency planning is a preventative measure that involves selecting the optimum set of transmitter and receiver frequencies at a collocated site such that the resulting IM occurrences in the receive band are at a minimum. Software tools to perform IM analysis are available readily and can be used to predict the IM products given the set of transmitter and receiver frequencies.
Antenna isolation through spatial separation is possibly the most flexible and versatile collocation parameter, especially if the collocation interference analysis is performed at system build out when equipment modification is a last-resort option. Unlike other measures, antenna isolation combats all types of interference mechanisms and, in addition, is independent of the base station equipment. Antenna isolation is primarily a function of the spatial configuration and the mounting structure. In the case of duplexed antennas, the duplexed transmit and receiver antennas are isolated by a fixed amount by means of a duplexer provided in the base station hardware.
Assuming omni dipoles, the free space antenna isolation values for a given configuration, as shown in Figure 6, can be estimated using
where
Lv = vertical isolation5 in dB
Lh = horizontal isolation (Friis transmission formula) in dB
Le = echelon isolation (linear interpolation between Lv and Lh as a function of angle) in dB
h = vertical tip-to-tip separation
(same units as d and l)
d = horizontal separation (same units as h and l)
l = wavelength
(same units as h and d)
tanñ1 (h/d)c = arc tan in radians
Gt,Gr = effective antenna gains in dBi
(rated gain is achieved only in the far field)
These approximations are used commonly in the industry. However, a more rigorous theoretical treatment exists.4,5 The antenna types (omni vs. panel), characteristics (downtilt or pattern) and relative configurations together with the mounting structures will influence the isolation values. The exact value can be determined only through actual field measurements, but the equations are useful tools in making preliminary assessments.
Figure 7 shows the variation of antenna gain with distance from the antenna. The measurements were made using a Sinclair SRL-480 10 dBd gain antenna at 850 MHz. The rated (constant) full gain of an antenna is realized only in the far-field or Fraunhofer zone. In the near-field or Fresnel zone, the gain increases with distance. The boundary between the two regions is at distance R from the antenna, given by
where
R = distance from the antenna
(near-field, far-field boundary)
L = length of the antenna
l = wavelength
Transmit filters are used to reduce the transmitterís out-of-band emission, thus reducing the broadband noise. The filter may be a bandpass cavity filter and/or a notch filter depending on the requirements. Filter performance is specified normally by means of a network analyzer swept over a frequency range. This plot can be used to determine the filter attenuation of the transmit signal at the frequency of interest.
In addition to suppressing image signals, the receive filters must suppress any collocated (out-of-band) transmitter signals that can desense the receiver front end and attenuate the levels of spurious signals that combine in the receiver to produce IM products. Reducing the levels of the spurious signals reduces the IM levels, thus providing the receiver with a measure of immunity to IM. 
Transmit isolators are used to reduce the level of the IM products at the transmitter amplifier, preventing closely spaced signals from other transmitters from leaking in and mixing in the transmit amplifier. The isolators are located at the output of the transmit amplifier, as shown in Figure 8, and attenuate signals in the reverse path that leak into the transmitter output. Consequently, any IM produced in the transmit amplifier as a result of these leakage signals is also attenuated. The isolator bandwidth, loading, impedance matching and harmonic filtering have to be considered carefully, otherwise the isolator may prove to be an additional source of IM.
An antennaís passive IM (PIM) performance dictates the levels of IM generated at the antenna. Receive antenna IM products (due to signals from collocated transmitters falling on the receive antenna) that lie in the receiverís in band cannot be filtered. Their severity can be controlled only through adequate antenna isolation and by specifying the antenna PIM performance.
Analysis Procedure
An analysis can be performed to determine either the equipment requirements or antenna isolation provided the other is known. Essentially, the procedure is the same in both cases.
Assuming a certain minimum antenna isolation is achievable, the required equipment performance specifications can be determined, including the transmit and receive filters, the transmit isolators and the antennas. This approach is useful during the system/equipment design and in special collocation situations where antenna spacing constraints require additional filtering/isolation hardware.
Assuming that the equipment (filters, isolators and antennas) performance specifications have been defined, the required antenna isolation can be determined and is used then to determine the antenna spacing and configuration requirements. This approach is useful at the deployment phase when equipment redesign is not a viable option.
A procedure is described that is specific to a site, and the filter, isolator and antenna PIM calculations are specific to each transmitter/receiver at that site. The first step is to gather relevant information regarding the collocated systems, including the maximum allowable interference noise level calculated from the receiver sensitivity and the maximum allowable degradation (receiver desense requirement). Transmitter data must be determined, including frequencies, bandwidths, power levels, amplifier broadband noise plots, conversion (mixing) loss, combiner loss, antenna cable loss and filter characteristics if antenna isolations are to be determined. The receiver data include frequencies, bandwidths, sensitivity, spurious levels, IP3 or IM specifications, muticoupler loss, antenna cables loss and filter characteristics if antenna isolations are to be determined. Finally, antenna data are determined, including antenna type, gain as a function of distance, PIM specifications if isolations are to be determined, isolations or relative configurations if equipment specifications are to be determined.
An IM analysis tool can be used to optimize the frequency plan for the minimum number of IMs and to predict the IM products. The number of IMs and the worst-case IM, in terms of IM component signal strengths, at each of the transmitters and receivers are selected as the basis for the analysis. In most cases, the analysis is restricted to the third-order IMs unless the number of fifth-order IMs is large. Typically, a fifth-order IMís level is 15 dB below that of a third-order IM for the same signal components.
The out-of-band emission from each transmitter is subject to several stages of attenuation before it reaches the receiver, including the bandpass filter following the amplifier; the combiner, if present; the transmit cable; the isolation between the transmit and receive antennas; the receive cable; and the receiver multicoupler, if present. The resulting broadband noise is the sum of the broadband noise at the receive antenna from all the transmitters at the site. The level of this noise should be less than the maximum allowable interference level, and can be represented by the following generalized equation that must be satisfied by the filter and antenna isolation for each transmit/receive pair:
TX broadband noise at RX frequency (dBm) ñ TX filter attenuation at RX frequency (dB) ñ feeder loss (dB)
ñ antenna isolation (dB) < threshold
(dBm) ñ number of TX (dB)
(11)
where
TX = transmitter
RX = receiver
feeder = combiner + TX cable + RX cable + multicoupler
The receive filter and the corresponding antenna isolation must satisfy two requirements based on the receiver desense requirement and on the receiver IM requirement. The worst-case result is then used as the applicable collocation parameter.
For the desense requirement, Equation 12 must be satisfied by the receive filter and the antenna isolation, and can be solved for whichever is the unknown. The equation is obtained by equating the (spurious) collocated transmit carrier level at the receiver to the maximum level allowed in order that the receiver desense threshold is not exceeded. The carrier signal from each transmitter is subject to several stages of attenuation before it reaches the front end, including the combiner, if present; transmit cable; isolation between the transmit and receive antennas; receive cable; preselector filter after the receive antenna; and multicoupler, if present. The level of this signal should be less than the maximum allowable level, represented as
TX level (dBm) ñ feeder loss (dB)
ñ antenna isolation (dB) ñ RX filter attenuation (dB) < threshold (dBm)
ñ number of TX (dB)
(12)
For the IM requirement, Equation 13 must be satisfied by the receive filter and the antenna isolation, and can be solved for whichever value is unknown. The equation is obtained by equating the level of the IM generating spurious signal strength to the threshold adjusted for the total number of IMs, expressed as
TX level (dBm) ñ feeder loss (dB)
ñ antenna isolation (dB) ñ RX filter attenuation (dB) < threshold (dBm)
ñ number of IM (dB)
(13)
Equation 14 must be satisfied by the transmit isolator and the corresponding antenna isolation, and can be solved for whichever value is unknown. The equation is obtained by equating the worst-case transmit amplifier IM signal level at the collocated receiver to the maximum level allowed in order that the receiver desense threshold is not exceeded.
In a typical scenario, a transmit signal leaks into another transmit path after attenuation in the combiner, transmit cable and antenna isolation. The signal undergoes further attenuation by the cable, combiner, isolators and filters, then mixes in the final amplifier stage generating IMs that are then reradiated along with the desired transmit signal. These IMs reach the receiver after undergoing attenuation due to the transmit filters, transmit and receive cable losses, and antenna isolation. The IM magnitude is determined by the magnitudes of each of the mixing signals at the point of mixing where the IMs are produced. The level of this noise should be less than the maximum allowable interference level represented by
TX1 level (dBm) ñ feeder1 loss (dB)
ñ antenna isolation (dB) ñ TX2 filter attenuation at TX1 frequency (dB)
ñ isolator loss (dB) ñ conversion loss (dB) ñ TX2 filter attenuation at IM frequency (dB) ñ feeder2 loss
ñ TX2/RX antenna isolation (dB)
< threshold (dBm)
ñ number of IM (dB)
(14)
where
TX1 = transmitter 1
TX2 = transmitter 2
feeder1 = combiner + TX1 cable
+ TX2 cable
feeder2 = combiner + TX2 cable
+ RX cable + multicoupler
Also, IM generation in the transmit amplifier can occur with any combination of local and leakage signals. Equation 14 will need to be adapted for the particular IM under study. The worst-case IM should be used in the calculations to determine the minimum required isolator and antenna isolation.
Equation 15 must be satisfied by the antenna PIM level and the corresponding antenna isolation, and can be solved for whichever value is unknown. The equation is obtained by equating the worst-case antenna PIM signal level at the collocated receiver to the maximum level allowed in order that the receiver desense threshold is not exceeded, expressed as
TX level (dBm) ñ feeder loss (dB)
ñ ½antenna PIM performance (dB)½
ñ antenna isolation (dB) < threshold (dBm) ñ number of IM (dB)
(15)
An Example
This example considers collocated Advanced Mobile Phone Service (AMPS) and narrowband personal communication services personal air communications technology (pACT) systems. The relevant system characteristics are listed in Table 2. The values listed in Tables 3 and 4 are typical of both systems. These values, together with the analysis equations, are used to obtain the equipment requirements listed in Table 5. The antenna isolations are assumed to be 33 dB. The antenna configurations consist of nonduplexed transmit and receive antennas in each system spaced such that every transmit/receive pair has a minimum isolation of 33 dB.
Assuming that frequency planning results in a worst-case seven IM hits in an AMPS receive channel and two hits in a pACT receive channel, the worst-case frequency separation for the pACT transmit is the pACT receive 39 MHz away. Solving Equation 11 for the pACT transmit filter attenuation at 39 MHz offset gives
pACT TX filter attenuation at 39 MHz
> (47 ñ 109.8) ñ (0 + 2 + 2 + 0) ñ 33
+ 137 + 12 > 49.2 dB (16)
The worst-case frequency separation for the AMPS transmit is the pACT receive 7 MHz away. Solving Equation 11 for the AMPS transmit filter attenuation at 7 MHz offset gives
AMPS TX filter attenuation at 7 MHz
> (47 ñ 90) ñ (4 + 2 + 2 + 0)
ñ 33 + 137 + 12 > 65 dB (17)
For the RX filters, the worst-case frequency for the pACT receive is the AMPS transmit 7 MHz away. Solving Equations 12 and 13 for the pACT receive filter attenuation at 7 MHz offset gives
pACT RX filter attenuation at 7 MHz
> 47 ñ (4 + 2 + 2 + 0) ñ 33 + 31 + 12
> 49 dB (18)
for the desense requirement, and
pACT RX filter attenuation at 7 MHz
> 47 ñ (4 + 2 + 2 + 0) ñ 33 + 48 + 3
> 57 dB (19)
for the IM requirement. From Equations 18 and 19, the applicable requirement is (Equation 19) based on worst-case considerations. The worst-case frequency separation for the AMPS receive is the AMPS transmit 20 MHz away. Solving Equations 12 and 13 for the AMPS receive filter attenuation at 20 MHz offset gives
AMPS RX filter attenuation at 20 MHz
> 47 ñ (4 + 2 + 2 + 4) ñ 33 + 31 + 12
> 45 dB (20)
for the desense requirement, and
AMPS RX filter attenuation at 20 MHz
> 47 ñ (4 + 2 + 2 + 4) ñ 33 + 48 + 8.5
> 58.5 dB (21)
for the IM requirement. From Equations 20 and 21, the applicable requirement is (Equation 21) based on worst-case considerations.
In solving for the isolator parameters, the pACT transmitter is a single-frequency transmitter. The worst-case IM results form the leakage of AMPS signals from the collocated AMPS transmitter into the pACT transmitter, creating IM in the AMPS receive channels. Using the pACT filter performance determined previously as a minimum, Equation 14 is solved for the pACT isolator reverse loss as
pACT isolator reverse loss
> 47 ñ (4 + 2 + 2) ñ 33 ñ 49.2 ñ 5
ñ 49.2 ñ (0 + 2 + 2 + 4)
ñ 33 + 137 + 8.5 > 7.1 dB (22)
Since the AMPS transmitter is a multiple-frequency transmitter, the worst-case IM results from the leakage of AMPS signals in the AMPS transmit combiner, creating IMS in the pACT receive. IMs in the AMPS receive, even though greater in number, are more attenuated by the transmit filter. Using the pACT filter performance determined previously as a minimum, Equation 14 is solved for the AMPS isolator reverse loss as
AMPS isolator reverse loss
> 47 ñ (4 + 0 + 0) ñ 0 ñ 65 ñ 5 ñ 65
ñ (4 + 2 + 2 + 0) ñ 33 + 137 + 3
> 7 dB (23)
In the antennaís case for the pACT system, the worst-case IM falls in the pACT receive channel and is generated by the collocated transmit signal falling on the pACT receive antenna. Solving Equation 15 for the pACT antenna PIM performance gives
½pACT antenna PIM½
> 47 ñ (4 + 2 + 2) ñ 33 + 137 + 3
> 146 dBc (24)
Note the level of the IM generating signal at the pACT antenna is +8 dBm.
For the AMPS case, the worst-case IM falls in the AMPS receive channel and is generated by the collocated transmit signal falling on the AMPS receive antenna. Solving Equation 15 for the AMPS antenna PIM performance gives
½AMPS antenna PIM½
> 47 ñ (4 + 2 + 2) ñ 33 + 137 + 8.5
> 151.5 dBc (25)
Note the level of the IM generating signal at the AMPS antenna is +8 dBm.
Conclusion
RF interference issues at a collocated site have been examined, and a general procedure and example to determine measures to combat this interference have been presented. A variety of system architectures and collocation configurations exist, and a comprehensive treatment would only render the study intractable. However, an understanding of the interference mechanisms and the procedures will help in extending the analysis to any collocation scenario. n
References
1. S. Haykin, Communication Systems, Wiley-Eastern, 1979, p. 297.
2. R.C. Sagers, ìIntercept Point and Undesired Responses,î IEEE Transactions Veh. Tech., Vol. VT-32, No. 1, February 1983.
3. ìIntermodulation Interference in Radio Systems,î Technical Reference, AT&T Bell System, November 1972.
4. J.D. Kraus, Antennas, Chapter 10, McGraw-Hill, 1988.
5. K.J. Affanasiev, ìSimplifications in the Consideration of Mutual Effects between Half-wave Dipoles in Collinear and Parallel Orientations,î Proc. IRE Waves and Electrons, September 1946.
6. Product Selection Guide 195, Celwave, Marlboro, NJ.
