Waste Figure and Waste Factor: New Metrics for Evaluating Power Efficiency in Any Circuit or Cascade

Today, there is a great need for ensuring the energy-efficient design of circuits and systems, yet the electrical engineering field has lacked a clear, well-defined, unified key performance indicator (KPI) for quantifying the power efficiency of any device or cascade of devices or systems. The industry has such a metric for quantifying additive noise along a cascade and this is known as the noise factor (F) or noise figure (NF) when expressed in dB. This standardized approach to quantifying additive noise now dons the specification sheets of virtually all receiving devices or receivers and is used as a key figure of merit (FoM) in the research and design communities when creating new devices or exploring system concepts.

This article develops a FoM for the comparison of wasted power along a cascade. This new FoM, called the power waste factor, or simply the waste factor (W) or the waste figure (WF) expressed in dB, is derived using a similar mathematical modeling approach taken by Harald Friis in 1944 to create noise figure. This article will show that the waste figure is a useful FoM for comparing and contrasting the power efficiency of any circuit or cascade of circuits or systems. Just as noise figure characterizes the additive noise of a cascade, waste figure characterizes the additive wasted power along a cascade. Waste figure is a handy metric to determine and compare consumed power along a cascade and is useful in identifying design choices that optimize power consumption while providing a measure of the power efficiency of any circuit or cascade. In an era where energy efficiency is more important than ever, the waste factor and waste figure are new metrics for accomplishing power-efficient circuits and system designs. The waste factor (W), also called waste figure (WF) defined as WF (dB) = 10 log (W), merely requires knowledge of the device efficiency and device gain. Following the mathematical modeling approach taken by Harald Friis where he developed noise figure,¹ this article offers a standardized framework for evaluating power consumption in any wired or wireless device, system or network. This framework may have utility in standards bodies to reduce global power consumption.

The waste figure provides a simple analytical formulation and mathematical model for the additive power wasted along virtually any cascade. As shown here, W enables extremely general analysis, with application to circuits, transceivers, channels and data centers. Virtually anything that forms a cascade of devices or systems for information flow may be characterized and quantified by W. Using the mathematical formulation of W in circuit and system analysis, it becomes possible to characterize specific power efficiency performance levels while gaining insight into design approaches that assure minimal power consumption. Furthermore, W allows a standardized way to interpret and analyze power consumption in any device or cascade, making it a powerful analysis tool as well as the basis of a learning model for artificial intelligence (AI) and machine learning (ML) design and control for optimized power efficiency. While some authors have recently applied the waste figure to analyze the power efficiency and to make design tradeoffs in unmanned aerial vehicle (UAV) cellular infrastructure systems,² millimeter wave wireless network³ phase shifters in sub-THz phased arrays and data centers,⁴ the waste figure remains relatively unknown and undiscovered. However, it offers a standard FoM for evaluating the power efficiency of any circuit or cascade.

This paper introduces the mathematical derivation of the waste figure and shows that its mathematical basis is strikingly similar to noise figure. The definition of waste figure is a simple and powerful metric based on the efficiency (η) and gain (G) of the various elements of a cascade. While the power efficiency may be referred to either the input or output signal power of the cascade, it is most sensible to consider the waste factor as related to the signal output power of a device or cascade, P_signal,out. The result of the waste figure for a cascade will be derived and it will be shown that the mathematical expressions for the waste figure of a cascaded system have a nearly identical form to Friis’ original noise figure expression. The canonical results when deriving the waste figure for a passive load will be shown, which yields mathematically similar results as compared to the noise figure as well as key results when using W to analyze the power efficiency of a transmitter (TX)-receiver (RX) link with a lossy channel as part of the cascade. It will be shown that the waste figure may be used in circuit design to indicate the most power-efficient cascades while illuminating the most critical components that dominate power consumption and thus energy efficiency.

As shown subsequently, waste factor denotes the total power consumed by a circuit or cascade, divided by the signal power out of the circuit or cascade, and represents a new way to consider wasted power or power efficiency along a cascade. By definition, W is always greater than or equal to 1, where W=1 (WF=0 dB) denotes an optimally power-efficient circuit or cascade, where all the power consumed by the circuit or cascade is found to be in the usable signal output power. Conversely, if W equals infinity, it means there is no power output delivered from the cascade while power is being consumed (e.g., a dummy load). The article shows that waste factor is the inverse of power efficiency in standard passive circuit theory and the inverse of the original definition of total power added efficiency (PAE) for DC-powered amplifiers.¹² The waste factor may be used to readily quantify the total wasted power and efficiency of a cascade of matched, linear devices.

Examples will be shown to illustrate how waste figure can characterize the power efficiency of various types of cascades, including a cascade of a TX, a propagation channel and a RX, a homodyne transmitter, and even a data center (we show here how W offers improvement over a popular data center power efficiency metric). These examples allow engineers to make simple approximations regarding the impact and placement of power-efficient components in a radio network operating in a lossy channel. The virtue of using W is that a standard metric may now be used to demonstrate intuitive design choices. This will allow engineers to conduct power efficiency studies on a circuit or cascade, or any type of source-to-sink link.

FUNDAMENTALS OF NOISE FIGURE (NF) AND WASTE FIGURE (WF)

This section shows the duality between the derivations of noise figure (NF) and waste figure (WF), which are used to evaluate additive noise and additive wasted power in any circuit or cascade, respectively.

Noise Factor (F): Quantifying Additive Noise in Cascades

Noise factor (F) defines the additive noise along a cascade which causes degradation of SNR along the cascade. The noise factor, F, was defined by Harald Friis in 1944¹ as the ratio of the input SNR to output SNR, where F=SNR_i/SNR_o. When F is expressed in dB terms, it is referred to as the noise figure, where a value of 0 dB indicates that no added noise and no degradation in SNR occurs along a device or a cascade of devices. Friis’ formula is widely used to calculate the overall F of cascaded devices, where each device has its own individual F and power gain, G. Once the total F is calculated for a cascade, it can be used to determine the overall noise power contribution of the entire cascade.

Friis was interested in modeling how the input noise level would be amplified and intensified in a receiver and created a mathematical model that considered the additional noise power contributed by components moving from the source (input) to the sink (detector). He was aware that there existed a nominal input thermal noise level to the cascade of kTB that could be measured as well as an output noise level that could be measured. Thermal white noise power is defined by: N=kTB, where N is the noise power available at the output of a thermal noise source, k=1.380×10^-23 J/K is Boltzmann’s constant, T is the temperature in Kelvin and B is the noise bandwidth in Hz. At the input of a cascade, Friis was able to turn a signal on and off that could be added to the noise and detected at the output of the cascade. In this manner, he was able to adjust and measure the SNR at the input to the cascade relative to the output of the cascade. Since he had control of the cascade input signal power level and could measure the noise power at the output of the cascade relative to the signal and noise powers at the input, he defined noise figure relative to the input noise power of the cascade as shown in Equation 1:

From Equation 1, F for a cascaded system with matched loads is given by Equation 2, where the first component F₁ is closest to the source, (e.g., the antenna or front-end of a RX). Friis found Equation 2 simply by systematically applying Equation 1 at each successive stage in a cascade realizing that each component had output noise N_o which included an additive noise component related to F.

In Equation 2, F_i represents the noise factor of the i_th device and

represents the power gain of the i_th device (linear, not in dB). For a given noise input power, N_i and noise output power, N_o, Friis showed that F (defined in Equation 1) was directly related to the additive noise contributed by the device or cascade alone as defined in Equation 3:

From Equation 3, it is clear that (F-1)GNi represents the additive noise power contributed by the component or cascade, above and beyond the input noise power and as referred to the input. F = 1 (0 dB) means there is no additional noise power contributed by the cascade other than what was applied to the input and then amplified with ideal noiseless amplifiers.

Waste Factor (W): A New Metric for the Analysis and Comparison of Power Efficiency

Waste factor (W) or waste figure (WF in dB) characterizes the power efficiency of a cascaded system by modeling the power wasted (e.g., power consumed but not delivered as output power) in each of the components along a cascade. Like the mathematical modeling method used by Friis to derive noise figure, the power wasted by a device or cascade may be analyzed by modeling the progressive useful signal power transferred along a cascade relative to the total power consumed by the components of the cascade. The derivation of the waste figure assumes that all power that is not transferred along the cascade as signal output is simply “wasted power” since such wasted power is not being passed along the cascade to the next stage as a useful signal.

This is a new way of defining power efficiency. In this way, it becomes clear that instead of additive noise being accumulated at each stage of a cascade as in noise figure, the amount of wasted power (e.g., power not delivered in the signal to the successive stage) from each of the components accumulates from the input to the output. Since it is easy and customary to measure the power consumption of an entire cascade of devices (e.g., the total power consumed by a system) and also easy to measure the signal power at the output in watts (e.g., P_out = GP_in), the waste factor is defined relative to the output of a device or cascade and is given by Equation 4:

Where:

η_w = waste factor efficiency and it equals 1/W

P_consumed = total power consumed by the device or cascade

P_out = output signal power

P_non-signal = total consumed power minus the signal power delivered to the output, which is the wasted power of the device.

As mentioned previously, the waste factor for an active component is the inverse of the total PAE, a key metric for assessing power amplifier efficiency as shown by A. A. Sweet.¹² The original PAE metric quantifies how effectively an RF amplifier with a DC power supply transforms the total consumed power, encompassing both the DC power supply and RF input power, into RF output power and is defined as¹²

Inspection of Equation 4 (also see Equation 11) shows PAE^#1 is identical to the reciprocal of W. In 1993, Walker¹³ introduced a second definition of PAE, given as

which is now widely regarded as the industry standard for evaluating the efficiency of RF power amplifiers. It is easy to show from Equation 4 that W is related to PAE^#2 such that

which is nearly identical to PAE^#1 when gain is large.

Similar to how Friis defined noise figure as NF = 10log₁₀(F) (dB), the waste figure is defined as WF = 10log₁₀(W) (dB). It was shown^3,4,7,14 and derived previously that the waste factor of a cascade is expressed as Equation 5:

In Equation 5, W_i represents the waste factor of the i_th device and

represents the power gain of the i_th device (linear, not in dB) and the N_th device is the closest device to the information sink (e.g., the output of the cascade). Note the mathematical similarity between Equation 2 and Equation 5, except Equation 2 increases from source to sink and Equation 5 increases from sink to source.

For a given signal input power to a cascade, P_in, and cascade signal output power, P_out, W is a FoM that represents a measure of total additive power that is wasted (e.g., not part of the delivered signal power) at the output of a cascade. W quantifies the amount of consumed power that is wasted (e.g., consumed power that is not found in the output signal power) relative to the total signal power delivered at the output of the cascade, independent of the signal power applied at the input of the cascade (see Equations 8 and Equation 9). Thus, W defines a relationship between added wasted power of a device or cascade, vis à vis the delivered signal power in a similar way that Friis defined in Equation 3. This is shown in Equation 6:

From Equation 6, it is clear that (W - 1)P_out represents the total additive wasted power contributed by a component or by all the components within the cascade and is conveniently referred to the output. W=1 (0 dB) means there is no wasted power contributed by a component or by the cascade and the cascade has perfect 100 percent efficiency with all the power consumed by the cascade found in the output signal power. Similarly, W = infinity means all the power is wasted in the cascade, with no output signal power. Such formulation provides an intuitive way to understand power waste at each stage of the cascade and allows engineers to use W to compare the power efficiency of different devices and systems in a new way, just as noise figure did for noise analysis 80 years ago.

Figure 1 Power decomposition of a cascaded communication system or device.^5-7

The mathematical basis of waste factor and waste figure was derived originally by Murdock and Rappaport^5,6,7,14 by noting that the power consumption of any device in a circuit or system may be decomposed into four distinct power consumption components which may be summed together to determine the total consumed power of any device or network. The four power components include (i) the signal path power delivered to the output by a device on the signal path within a circuit or cascade (e.g., the output signal power that is delivered to the sink), (ii) the power consumed by a device which is on the signal path (e.g., a component on the cascade), but which is not provided in the output signal path (this is called the additive “wasted” power of a component, since as described previously, the component is on the cascade but such power is not delivered to the output signal of the device), (iii) all other power that is consumed by components that are not on the signal path or which are not associated with a device on the signal-path cascade and (iv) the signal power delivered to the input of the device or cascade by a source that is connected to the input. Figure 1 and Table 1 describe the four types of power that make up any circuit or system.

The non-path powers are not considered in the derivation of waste figure since these components are not found in a cascade along a signal path, just as noise figure does not consider non-path components as noise contributors. However, the total power consumption of both the signal path and non-path components is easily found using W and the power components of Table 1 using upcoming Equation 9. While waste figure characterizes a device or cascade on a signal path, the basic principles of power superposition and waste factor may be useful in analyzing non-path wasted power and power consumption using similar mathematical models and new figures of merit. It may be that non-path powers include the quiescent power drain of cascaded components that are in a sleep/off state or for the case when impedances are high-Z or greatly mismatched. This remains an open area for definition and usage.

In the development of noise figure, the output power of the input source was due to thermal noise at the receiver input. For waste figure, the output power of a signal source is the signal input power to the cascade or device. When considering the four power sources of Table 1, it becomes clear that for any cascade or device along the signal path, which will be called a “system,” the total power consumed by just the system carrying the signal (P_consumed), is equal to the total power consumed by the system, including the input signal power, minus the input signal power to the system, in order to refer W to the output of the system alone, regardless of input signal levels (assuming linearity). This is expressed in Equation 7:

where P_system-added is the signal power added by the system and the signal output power of the system is P_signal = P_source,out + P_system-added. From Equation 7, the total power consumption of the system along the signal path, independent of the input signal power, P_source,out, is given by the superposition of Equation 7 and is shown in Equation 8:

The use of W in Equation 6 and Equation 8 provides a convenient method to compute the total and wasted power consumed by a device or cascade. Using Equation 8 and then by including the non-path powers, P_non-path, contributed by all components that are off the signal cascade (such as displays, heat sinks, power supplies, etc.), the total power consumption of any system may be found as shown in Equation 9 using superposition of all power types in Table 1:^7,14

The only factors that must be known for every device on the signal path along the chain of a cascaded network are gain and efficiency. Gain has the standard definition for the signal path components in the cascade that carry information to later stages of the cascade and the efficiency is defined as the reciprocal of the waste factor (W) in Equation 4. These two formulations are shown in Equation 10:

As noted earlier, the waste factor efficiency, η_w, as defined in Equation 4 and Equation 10, is the reciprocal of W. For passive devices, 1/W = η_w is identical to the traditional definition of efficiency when relating output power to input power

In the special case of active components powered by a DC source, W is equal to

which is equal to the reciprocal of PAE^{#1 12} as shown in Equation 11 from Equation 8:

RF, microwave and circuit engineers characterize amplifiers using PAE.^10-13 While Equation 11 shows total PAE¹² (PAE^#1) is the inverse of the waste factor for the case of an amplifier that uses a DC power supply, a more customary definition for PAE,¹³

is easily shown to be related to W as:

which is nearly identical to Equation 11 when G is large. To consider the power wasted along a cascade of active devices, the reader can use Equation 5 which is proved in Equations 22 to 24 by simply using to characterize power efficiency and power consumption using the waste factor of a cascade, similar to how noise factor is used to quantify additive noise and SNR degradation for a cascade.

It should be clear that waste factor efficiency accounts for the proportion of signal power output when compared to the total power consumed, on the signal path cascade where the total power consumed includes both output signal power and all non-signal (e.g., wasted) power, independent of the input to the device or cascade. From Equations 4, 8 and 10, it can be seen from superposition that P_non-signal (additive wasted power in a device or cascade) and the total power consumed by the device or cascade are related to W by Equation 12 and Equation 13:

Fundamentally, Equation 4 and Equation 10 show that W is defined as the inverse of efficiency.^3-7 Defining the waste factor in this manner provides a FoM that may be used for any device or cascade and relates the waste factor to additive wasted power, just as noise figure did for additive noise power. This is shown in Equation 14.

Because W characterizes the useful signal power delivered to the output of any device or cascade relative to the entire power consumed along the device or cascade, it is most sensibly calculated referred to the output. Employing the concept of waste factor allows one to quantify power dissipation within a device (e.g., wasted power, since some power is not contained in the signal that is carried forward) which from Equations 13 and 14 and Table 1 can be expressed in Equation 15:

Figure 2 A general cascaded communication system with a pair of devices.

It is clear from Equation 15 that the input power to the cascade, (e.g., P_source,out) is not required to be known when using W, since W is a characteristic of the cascade or circuit, unrelated to input or output signal powers, as the cascade is assumed to be linear and matched. When a device along the cascade is turned off (e.g., P_signal = 0 or P_source,out = 0), W is still defined for a device or cascade, just as Friis was able to turn off the input signal power and still define F for a cascade, even when F = SNR_i/SNR_o was infinite or undefined.

The formulation of W for a cascaded system as shown in Equation 5 is proved using the simple cascade of two devices shown in Figure 2.

Using Figure 2, the waste factor for the cascade signal path (where P_non-path from auxiliary components is neglected since they are not on the cascade) can be calculated as illustrated using a cascade of two devices. First, the total power consumed at the output terminal of network 2 can be defined using Equation 4 or Equation 10 as shown in Equation 16:

Then, the power consumed at the output terminal of network 1 can be defined in Equation 17:

Here, P_consumed,1 denotes the total power consumption at the output terminal of device 1, which includes both the signal power applied to the input as well as the additional power consumed and contained in the signal that is transmitted to the subsequent device. It also includes the power wasted (e.g., not contained in the signal) by device 1, itself. When P_source,out (the input signal power) is subtracted from the total consumed power using Equations 7, 16 and 17, the standalone power consumption of device 1 is defined by Equation 18:

Applying the same approach to device 2, the standalone power consumption of device 2 is defined in Equation 19:

Intuitively, the total power consumption of the cascaded system of the two devices is the sum of power consumed by each device alone and the power input to the system and this is shown in Equation 20.

Also, the output signal power of the cascade is shown in Equation 21:

It follows from Equation 18 and Equation 19 that the total power consumption of the cascade can be shown in Equation 22:

Since Equation 22 is of the same form as Equation 13 and Equation 8, the power waste factor for the cascaded system of Figure 2 is given by Equation 23:

From Equation 23, W for a cascaded system with N devices may be generalized in Equation 24:

Note the approach and mathematics are strikingly similar to the cascaded noise factor in Equation 2,¹ except W is referred to the output and is generally most impacted by the component closest to the Nth component closest to the sink.^5-7

The wasted power of the cascade when there is a continual signal flow can be determined based on either the output power as in Equations 6, 8, 12 and 15 or based on the input source power and the gain of each stage as in Equation 25, but a signal flow to the output is not required to define W.

In Equation 25, W is the waste factor for the entire cascade and G_i is the gain of i_th stage, with i = 1 denoting the stage closest to the source and N is the number of cascaded components.

HANDLING TIME-VARYING POWER STATES WHEN USING WASTE FACTOR

In a cascaded system, the components often exhibit dynamic behavior of varying power efficiency, leading to temporal variations in the waste factor. Amplifier efficiency levels may change above their quiescent power consumption levels over time when a signal of varying magnitude is applied or different devices, systems or chains are turned on and off at different times or for different functions to conserve power, etc. To account for these fluctuations, it is practical to calculate the average waste factor of each device over a designated time interval or averaged over all the finite power states. This can be mathematically described using the time average, which is simply the integral of the time-varying waste factor over an observation interval. Just like with the noise factor, a time-averaged waste factor may be used, denoted as __W in Equation 26:

Where:

T represents the selected period over which the average is taken, presumably over all operating states

W_t denotes the instantaneous waste factor at time t

W_i represents each of the finite number of power states

In this calculation, there are N states and the duration of each state is considered in the average. This formulation provides a time-averaged measure of the waste factor to capture an average value of the dynamic nature of the power consumption and efficiency. For on/off components (e.g., amplifiers) with static/quiescent power, when a state is “off” during an observed epoch, the static power of such components may be treated as non-signal power (P_non-signal), although it may be more useful to consider these “turned-off” devices as contributing to non-path power rather than using Equation 26 since the “turned off” components do not carry signal power across a cascade when in the off state. This is an open research area ripe for discovery and definition of convention.

ANALOGIES BETWEEN WASTE FACTOR AND NOISE FACTOR

The analogous mathematical formulation of F and W is immediately apparent from the above text. However, there are important characteristics of each metric to keep in mind. Since noise figure is a measure of the additive noise from a source that results in the degradation of SNR caused by the components in a cascaded system, it quantifies the amount of total noise added to the signal referred to the input of the cascade. Therefore, F increases when observed from device 1 (closest to the source) to N (closest to the sink). On the other hand, W is a measure of the additive wasted power consumed by a cascaded system and quantifies the amount of power consumed and wasted by the cascade compared to the total signal power that is transported to the output. Since W is defined as the ratio of the total power consumed by the cascade to the signal output power, W is referred to the output and increases (e.g., efficiency decreases) when observed from device N (closest to the sink) to device 1 (closest to the source).

A larger value of W signifies more power wasted. The value of W is always equal to or greater than 1, with W = 1 signifying that all power supplied to a component or cascaded network is fully utilized in the signal output. This is the optimal condition with no power wasted. W equal to infinity indicates that no power is contributed to the signal output and all power is squandered (e.g., a perfect dummy load or a completely lossy channel). Some comparisons in the utility and scope of F and W in communication systems are summarized in Table 2.

In conclusion, both F and W are useful in the analysis of communication systems. F is a well-established metric that provides a measure of the additive noise of a system and the degradation of the SNR. W is a new metric that provides a measure of the additive wasted power of a system and the energy efficiency of a system. Both metrics are important for circuits and communication systems, but as shown later in this article, W has further applications for understanding power efficiency in computing and processing, as well as communication systems. With the increasing importance of energy efficiency for our planet, W can be a useful metric for enabling the design of a wide range of greener systems.

APPLICATIONS OF WASTE FACTOR IN DEVICES AND SYSTEMS

Waste Factor for a Passive Device

Figure 3 Passive attenuator circuit diagram.

Consider applying the waste factor to an attenuating stage like the passive attenuator shown in Figure 3.

The gain of the attenuator is defined as the output power divided by the input power as shown in Equation 27:

Where:

V_i-1 is the input voltage of the attenuator

V_i is the output voltage of this attenuator

L is the loss of this component
(L > 1)

This assumes that the impedance at the input and the output of the attenuator are equal, which directly relates the power ratio to the square of the voltage ratio. Treating this attenuator as the i_th stage of the cascade, the output power is shown in Equation 28 as:

The power wasted by the attenuator (e.g., not provided in the signal output) is defined in Equation 29:

Where:

P_sigi is the total signal power delivered by the i_th stage to the (i +1)_th stage

P_non-sigi is the signal power used by the i_th stage component but not delivered as signal power

Based on the definition of W in Equations 4, 10 or 14, W for a passive attenuator is computed in Equation 30 as:

Note the duality between attenuators using the waste factor and the noise factor. Waste factor, represented by W_atten = L_atten, quantifies the wasted power expended in the attenuator, while noise factor F_atten = L_atten quantifies the degradation of the SNR due to additive noise.

Waste Factor for a Communication Channel

In a communication system, the concept of waste factor (W) provides valuable insights into comprehensively understanding the power efficiency of the data transmission process through a lossy channel.^5-7 Any type of channel, whether wireless, wired, optical, etc., plays a vital role in the overall power efficiency of an end-to-end communication link. Consider a scenario where the power transmitted from the source is denoted as P_TX and the power received at the receiver is P_RX. Consider a lossy channel with a loss (e.g., L = 1/attenuation) given by Equation 31:

Also, the channel gain can be expressed as the reciprocal of loss as shown in Equation 32:

Assuming the i_th stage of a cascaded system represents the channel, then the signal power out of the channel, P_signal,i, is derived in Equation 33:

Here P_signal,i-1 corresponds to the output power of the transmitter P_TX, P_signal,i equates to the signal power out of the channel and is applied as input power at the receiver, P_RX. Consequently, the non-signal power P_non-signal,i within the channel represents the amount of transmitted power not successfully received out of the channel (e.g., channel loss or path loss) which is additive wasted power of the channel which is a component of the cascade between source and sink and this can be formulated as Equation 34:

Figure 4 Homodyne transmitter with a matched load.

where (1-G_chan) quantifies the proportion of the signal power lost due to various factors such as attenuation, scattering and absorption. Multiplying (1-G_chan) by P_signal,i-1 yields the amount of power dissipated during transmission and is the power lost or wasted in the channel. The waste factor for the channel can readily be calculated using Equation 34 to get the results of Equation 35:^5-7

Notably, unlike electrical devices, a wireless channel does not have a non-path power component (P_non-path), making it like a passive attenuator. Therefore, the waste factor for a channel (W_chan) is equivalent to that of a passive attenuator (W_chan=L_chan).

Waste Factor for a Homodyne Transmitter

The section presents a waste factor application example to compare two cascaded systems with different components. We compare the power efficiency of two different homodyne transmitters, each with matched load termination at the antenna. Figure 4 shows the homodyne transmitter block diagram.

Table 3 illustrates the application of the waste factor (W) and the value of W for a cascade as described in Equation 5 and Equation 13 to evaluate the impact of overall energy efficiency using different components in a homodyne transmitter system.

Table 3 and Figure 4 are illustrative for how to use W to characterize energy efficiency based on the selection of different components. Using Case 1 as an example, the waste factor of the homodyne transmitter based on component parameters in Table 3 is calculated using Equation 5 or Equation 24 and W = 1/η_w.

Example 1 in Table 3 compares Case 1 and Case 2 to show how components with different efficiencies impact W of the cascade using Equation 5 or Equation 24. It can be seen that Case 1, with a more efficient RF amplifier, results in a lower W of 2.20 (3.42 dB), signifying better power efficiency and less wasted power than Case 2, which uses a less efficient RF amplifier and yields a larger W of 4.12 (6.15 dB) for the cascade. Example 1 shows that almost twice as much power is wasted in Case 2 (e.g., 6.15 dB – 3.42 dB = 2.73 dB) which is roughly the proportion of efficiencies of the two different final amplifiers. The above example shows that amplifier efficiency closest to the sink dominates the overall waste figure of the cascade.

In Example 2 of Table 3, Cases 3 and 4 are considered. Here, again, overall W, as computed in Equation 5 or Equation 24, is impacted by specific components, but for this example, we see that components farthest from the sink have much less impact on overall power efficiency. Case 3 shows how a baseband amplifier that is much more power efficient achieves only a slightly lower W of 3.39 (5.30 dB) compared to Case 4, which uses an identical RF amplifier but a much less efficient baseband amplifier. Case 4 has only 0.09 dB poorer energy efficiency with a W of 3.46 (5.39 dB). While these observations are intuitive, waste factor allows precise quantification and comparison.

These examples illustrate the importance of optimizing power efficiency in the components closest to the sink to reduce waste and enhance system efficiency. These examples also show how waste factor may assist researchers and engineers in creating energy-efficient component technologies and system designs.

Waste Factor for a Communication System with a Channel

Figure 5 A communication system including a channel.

Figure 5 shows a system with a channel that is easily treated as a cascaded system. Note that P₁ is the power from the TX, P₂ is the power out of the channel and into the RX, and W_TX and W_RX represent the waste factors of the TX cascade and RX cascade (not shown), respectively. Note that the channel may be wireless, wired, acoustical, optical, etc. and may have multiple users/links.

The total waste factor for the cascaded system, including the TX and RX components as well as the inherent loss of a channel, is defined as W_sys. Using Equation 5 or Equation 24, W_sys is given by Equation 36 as:

From Equation 35, it is clear that W_chan=L_chan and when the receiver gain, G_RX, is substantially less than the loss of the channel, L_chan, which is typical for wireless links, W_sys in Equation 36 then simplifies to Equation 37:^5,6,7

Equation 37 implies that to achieve a smaller W_sys value, which is indicative of a more power-efficient end-to-end system, it is essential to have high-gain receivers, and transmitters with high power efficiency.

The key result of Equation 37 may be written in dB as Equation 38:

Equation 38 shows that the strategic configuration of power-efficient TX output components and high-gain receivers minimizes energy waste over any channel, optimizing the system energy efficiency. The simplicity of the system depicted in Figure 5 underscores the need for certain components to be properly designed for greater power efficiency to have the greatest impact on the overall minimization of power waste and offers a framework for understanding mobile system power consumption for individual users.

Now investigate how waste figure varies for different communication systems comprising a simplified TX, a lossy channel and a RX. The assessment considers the system architecture of Figure 5 while varying system gain and efficiency at each stage as shown in Table 4. This comparison enables an intuitive understanding of how changes in system gain and efficiency impact the overall energy efficiency of the communication system in Figure 5.

The different cases in Table 4 illustrate that when the channel loss increases, as seen between Case 1 and Case 2, the waste figure increases by a similar amount (e.g, the channel loss dominates power efficiency). Case 3 shows that making the RX more efficient (e.g., increasing its efficiency by 25% from 0.8 to 1), does not significantly affect the waste figure, reducing it by only 0.02 dB. However, in Case 4, when the TX efficiency is improved (e.g. doubled) from 0.5 to 1 with all other parameters the same as in Case 3, there is a noticeable decrease of 3 dB in the waste figure, which is equal to the increase in TX efficiency. This design saves half the consumed power for the same link quality. Case 5 uses the setup of Case 3 with the RX system gain increased from 60 to 70 dB. This saves much more power with the waste figure dropping by 10 dB. Comparing Case 5 and Case 3, Case 5 is vastly more energy efficient. By increasing the RX system gain, Case 5 needs only one-tenth the TX power compared to Case 3 for the same cascade output power. This efficiency is shown by a 10 dB reduction in the waste factor, indicating that Case 5 uses energy 10 times more effectively than Case 3.

The conclusion from these observations is that if a lower loss channel is not available, increasing the RX gain has a significant impact on reducing waste. Additionally, improving the efficiency of the TX is also a vital way to make the system more power efficient. The waste figure theory was first tested for the power consumption tradeoff between a single hop communication system versus a relay system, whereby operating regions were found that indicated which architecture was more power efficient to use for an end user.⁷

Waste Factor Applied to Data Centers

Data centers are one of the largest consumers of power today. The information technology (IT) field has established the power usage effectiveness (PUE) FoM.⁸ Employing the PUE to determine the power usage of non-IT components in relation to servers in a data center offers a straightforward approach to associating non-IT power consumption with the power consumed by server operations.

The IT industry defines PUE as the ratio between the summation of the amount of energy consumed by IT equipment and the energy consumed by auxiliary equipment for data operations, compared to just the IT equipment energy usage. As shown in Equation 39, the IT equipment includes networking equipment that is on the signal path, (e.g., switches, routers, firewalls, etc.) as well as components that do not transfer data but that are vital to data processing and thus may be considered as being on the cascade (using the waste factor model of Figure 1) but as not contributing (e.g., wasted power in the transport of information in servers, storage systems, etc.). The energy consumption of auxiliary equipment includes cooling, lighting and non-network devices that do not carry data center information, etc., and are similar to non-path components in the waste factor model (e.g. see Equation 9)^4,8

Since a data center provides information (e.g., data) from a source to a sink in its operation, the waste factor can be applied to gain new and vital insights to optimize the data center power efficiency. W can be applied to a data center if certain assumptions are made about how and where the signal power is transferred. Assuming that Equation 39 defines P_IT as the power consumed by the IT equipment, (e.g., signal path power and wasted power on the signal path) and P_aux is the auxiliary equipment power consumption, then Equation 39 may be recast in terms of W. This use of W considers only the powers consumed and delivered from a source to a sink within a data center while treating P_aux as off-path power that is not involved in the computation of W per Equation 9. Total data center power consumption is then computed through the sum of signal-path and non-path powers.

In the data transmission or processing within a data center, the major power consumption is attributed to servers, network switches and computing equipment, while additional power consumption is associated with cooling systems, power distribution units (PDUs) and other auxiliary equipment. According to findings,⁹ servers and networking equipment account for about 60 to 70 percent of the overall power consumption in a data center. Cooling systems contribute about 30 to 40 percent of the total power consumption, while the remainder is consumed by power distribution units (PDUs) and other auxiliary equipment.

To break down the information path power consumption of the data center, the total data center information path power consumption is modeled in Equation 40:

where P_info is the sum of all powers of each component that is used for carrying information or data in the system. The information path power is the network within the data center (e.g., P_router, P_switch, P_firewall) and other network equipment that carry information. This is similar to the previous definition of signal powers stemming from components on the cascade and it is defined in Equation 41:

and P_non-info is defined as the power used by the other IT-critical components that process the data but that are not directly involved in data transmission (e.g., P_processor, P_memory, P_storage, P_NIC).This is similar to the previous definition of the non-signal or wasted power of cascaded signal path components. The power consumed by the non-info components is shown in Equation 42:

where NIC represents the network interface cards.

Using this dichotomy to represent a data center in terms of a fine-grain consideration of components, Equation 40 may be used to recast PUE, as defined in Equation 39, as Equation 43:

From Equation 39 and Equation 43, the data center’s total IT power consumption (e.g., analogous to total power consumed by the cascade) can be rewritten in terms of PUE and the useful and wasted powers on the signal path as Equation 44:

Now, just as in previous sections, based on Equations 14, 43 and 44, the waste factor of the data center (ignoring auxiliary power similar to ignoring off-path power) can be defined as Equation 45:

The total power consumption for the data center, like the approach in Equation 9, can then be calculated by considering the data center as a single system that has signal path components, with some that carry information and some that do not, as well as auxiliary non-path components. Using Equation 45, Equation 9 and the definition of PUE, the total consumed power is defined in Equation 46:

The interpretation for W in Equation 46 is intuitive as it is for circuits or communication systems and relates W to PUE, an existing FoM in data centers. However, this interpretation requires a finer definition of components that map to Figure 1, and this approach enables a better understanding of the power efficiency of the data transport.

Figure 6 Illustrative comparison of two data centers.

The example in Figure 6 shows how waste figure applied to a data center provides a more detailed understanding of power efficiency compared to the commonly utilized PUE metric in data center evaluations. Consider two data centers with equal PUE values but with different architectures.

The example of Figure 6 assumes that Data Center A is a larger facility with more equipment and a higher total energy consumption. Conversely, Data Center B is smaller and uses less total energy. Assuming both data centers have identical PUEs and comparing total energy use, it might seem that Data Center B is more efficient. However, PUE, like waste factor, is designed to determine relative or proportionate energy efficiency without respect to actual consumption levels.^8,9 Waste factor, with its focus on power wasted on the path that transfers data, provides a better measure of the power efficiency of these two data centers since their ultimate mission is to transfer data in a network. Table 5 shows a comparison of the power consumption on PUE for both data centers.

For Data Center A, the power allocation is as follows: P_info,A = 140 kWh for information transmission, P_non-info,A = 40 kWh for non-data transmission components and P_aux,A = 150 kWh for auxiliary equipment. In comparison, consider Data Center B, which allocates P_info,B = 60 kWh of power for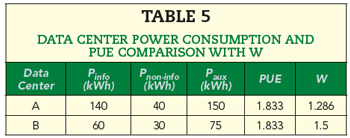information transmission components, P_non-info,B = 30 kWh for non-data transmission components and P_aux,B = 75 kWh for auxiliary equipment. This example has been specifically chosen to ensure the PUE values for each data center are identical (e.g., PUE would indicate they are equally energy efficient).

where PUE_A and PUE_B denote the PUE of Data Center A and Data Center B, respectively.

Now, using Equation 45, W for the data centers can be calculated:

By evaluating the waste factors, W_A and W_B, it is apparent that Data Center A is about 20 percent more efficient in its energy use in transporting data, even though both have identical PUEs. This efficiency is measured by comparing the amount of power used directly for carrying and processing information in the system to the overall power consumption. The PUE metric, although standard in the industry, does not capture the detailed energy usage of specific equipment and their relative power waste along the signal path, which is the ultimate job of a data center. It is worth noting that this result is somewhat analogous in Reference 3 where wider bandwidth THz channels are more power efficient on a per bit basis than narrower millimeter wave channels. The waste factor offers a more detailed perspective by considering the function and efficiency of individual components, which may be defined with their own W values. This analysis demonstrates the potential benefits of adopting the waste factor as a metric for evaluating energy efficiency in complex infrastructures such as data centers. Utilizing W as an efficiency metric can provide new insights into optimizing power consumption across various systems involving a source and a sink.

CONCLUSIONS AND FUTURE DIRECTIONS

The waste factor (W) or waste figure (WF in dB) is a new figure of merit for quantifying power efficiency and offers a useful advancement in the field of electrical engineering and system design. By providing a standardized metric for power consumption, it becomes possible for designers and researchers to have a common approach to quantifying power efficiency. This article has shown how the mathematical formulation is similar to the historical approach used to create the noise factor (F), yet W has much broader applications to systems of all types. The waste factor enables electrical engineers and circuit designers to quantify and minimize power waste in any circuity or cascade of devices or systems. This paper has demonstrated the foundational principles of the waste factor, its mathematical derivation and its practical applications across various scenarios, including passive devices, wireless channels, communication systems and data centers. More applications are possible.

Waste factor provides an intuitive understanding and mathematical formulation of power consumption within cascaded systems and allows for the optimization of designs in a manner that was previously challenging due to the lack of a unified metric. Moreover, the application of waste factor in emerging and critical areas such as UAV cellular infrastructure, millimeter wave wireless networks and data centers underscores its versatility and relevance in contemporary engineering challenges. As the demand for energy-efficient solutions continues to grow, the waste factor offers promise as a standard analysis tool for enabling green communications and sustainable technology development. Its adoption as an industry standard could drive significant improvements in the energy efficiency of future electronic devices and systems. The waste factor not only complements existing metrics such as the total PAE in amplifier design or PUE in data center design but also enriches the toolkit available to engineers for designing systems that are not only high-performing but also environmentally responsible. Future work may open new areas of application of the waste factor in both academic research and industry practice with the development of standardized measurement and reporting guidelines and applications of waste factor to other types of systems or devices. Additionally, further exploration of potential applications, such as in the use of AI and ML algorithms for power-efficient design, could lead to more innovative solutions and advancements in energy efficiency.

ACKNOWLEDGMENTS

The authors thank Nicola Piovesan, Antonio De Domenico and Prof. Hamed Rahmani for useful discussions, feedback and suggestions.

References

1. H. T. Friis, “Noise Figures of Radio Receivers,” Proc. IRE, Vol. 32, No. 7, pp. 419–422, 1944.
2. Y. Xing, F. Hsieh, A. Ghosh and T. S. Rappaport, “High Altitude Platform Stations (HAPS): Architecture and System Performance,” in 2021 IEEE 93rd Vehicular Technology Conference (VTC-Spring), April 2021, pp. 1–6.
3. O. Kanhere, H. Poddar, Y. Xing, D. Shakya, S. Ju and T. S. Rappaport, “A Power Efficiency Metric for Comparing Energy Consumption in Future Wireless Networks in the Millimeter Wave and Terahertz Bands,” IEEE Wirel. Commun., Vol. 29, No. 6, pp. 56–63, 2022.
4. M. Ying, D. Shakya, H. Poddar and T. S. Rappaport, “Waste Factor: A New Metric for Evaluating Power Efficiency in any Cascade,” IEEE Global Communications Conference, Kuala Lumpur, Malaysia, Dec. 2023, pp. 1–6.
5. T. S. Rappaport, J. N. Murdock and F. Gutierrez, “State of the Art in 60-GHz Integrated Circuits and Systems for Wireless Communications,” Proc. IEEE, Vol. 99, No. 8, pp. 1390–1436, 2011.
6. J. N. Murdock and T. S. Rappaport, “Consumption factor: A Figure of Merit for Power Consumption and Energy Efficiency in Broadband Wireless Communications,” IEEE GLOBECOM Workshops, pp. 1393–1398, 2011.
7. J. N. Murdock and T. S. Rappaport, “Consumption Factor and Power-efficiency Factor: A Theory for Evaluating the Energy Efficiency of Cascaded Communication Systems,” IEEE J. Sel. Areas Commun., Vol. 32, No. 2, pp. 221–236, 2014.
8. M. Dayarathna, Y. Wen and R. Fan, “Data Center Energy Consumption Modeling: A Survey,” IEEE Commun. Surv. Tutorials, Vol. 18, No. 1, pp. 732–794, 2016.
9. L. A. Barroso and U. Holzle, “The Case for Energy-proportional Computing,” Computer, Vol. 40, No. 12, pp. 33–37, 2007.
10. S. Lucyszyn, “Power-added Efficiency Errors with RF Power Amplifiers,” International Journal of Electronics, Vol. 82, No. 3, pp. 303–312, 1997.
11. M. Steer, “Microwave and RF Design V: Amplifiers and Oscillators,” 3rd ed., Vol. 5, NC State University, 2019.
12. A. A. Sweet, “MIC & MMIC Amplifier and Oscillator Circuit Design,” Artech House, 1990.
13. L. B. J. Walker, “High-Power GaAs FET Amplifiers.” Artech House, 1993.
14. T. S. Rappaport, “Waste Figure (W): A Figure of Merit for the Power-efficient (“Green”) Engineering of Any Device or Cascade of Circuits or Systems,“ ITU Webinar, Feb. 20, 2024, https://www.itu.int/en/journal/j-fet/ webinars/20240220/Pages/default.aspx.