Möbius Strips and Metamaterial Symmetry: Theory and Applications

Figure 1 Möbius strip (a) Möbius fly maze (courtesy of David Phillips)3 (b) Möbius strip made from silica (c).4
The inherent disposition of scientists and philosophers is to envision, to explore and to speculate about new things in this vast cosmos. The vastness of cosmos makes us believe that we live in higher dimensions, or Möbius-shaped universes, where one can traverse the entire path without crossing the boundary or edge, if any, with no ending.
For scientists, the Möbius surface is a topological exploration that exhibits unusual mechanical, acoustic and electronic properties to the limit that it violates the Hückel rules.1 Figure 1 shows the typical Möbius structure and defines the spatial relationships of shape that span dimensions in such a way that its metrical properties are barely changed (i.e., the properties of an object are unchanged when stretched or distorted). Some nanostructures have identical elastic properties.
A necessary and sufficient condition for a Möbius surface to develop is that its Gaussian curvature must vanish everywhere. In a curve with non-vanishing curvature, there exists a unique flat ruled surface (rectifying developable) on which this curve is a geodesic (see Figure 1a) described by Starostin and Heijden2,

where  is a parameterization of a strip with center line r, length L and width 2w; and where
is a parameterization of a strip with center line r, length L and width 2w; and where  is the unit tangent vector,
is the unit tangent vector,  the unit binormal, k the curvature and τ the torsion of the centerline. The parameterized lines s = const. are the generators, which make an angle β= arc [Tan(1/η)] with the positive tangent direction.
the unit binormal, k the curvature and τ the torsion of the centerline. The parameterized lines s = const. are the generators, which make an angle β= arc [Tan(1/η)] with the positive tangent direction.
As illustrated in Figure 1a, one can move along the length of a Möbius strip and return to its starting point having traversed the entire length of the strip without ever crossing an edge. In Figure 1b, find the path that the four flies take if they all travel the same route without meeting, and without retracing their path until they reach their original position. Keep track of which side of the path you are on.3 Knots using a miniature Möbius strip made from silica (see Figure 1c) are used for next generation liquid crystal (liquid crystal used in the flat panel displays on computers, TVs and smartphones all make use of its light-modulating properties).4
The unique properties of a Möbius strip results from the fact that its shape minimizes the deformation energy, which is entirely due to bending, described by

where D =2h3E/[3(1-v2)], where 2h is the thickness of the strip, and E and νare the Young’s modulus and Poisson’s ratio of the material.
A Möbius transformation f(z) is a fractional linear transformation to complex numbers:

From equation 4, the basic properties of Möbius transformations f(z) are described below: f(z) can be expressed as a composition of affine transformations (scaling: z → tz, translation: z → z +p, rotation: z → eiθz, complex conjugation: z →  , inversion: z →
, inversion: z →  ), where t, p
), where t, p 
 i, f(z) maps
i, f(z) maps  one-to-one onto itself, and is continuous; f(z) maps circles and lines to circles and lines; f(z) is conformal.
one-to-one onto itself, and is continuous; f(z) maps circles and lines to circles and lines; f(z) is conformal.

Figure 2 Proposed Möbius style National Library building in Kazakhstan.5
Equation 4, a powerful transformation, develops the Möbius surface as a topological structure that thus far has been limited to mathematical imagination. It can now be realized using metamolecules of different designs, enabling linear time algorithms for selecting optimal transformation, mapping, mesh generation, image processing, cryptographyand other branches of engineering useful in the physical world and day to day life.
Theory & Applications
Möbius Strips - Building
Figure 2 depicts an award winning building based on principles of the Möbius strip.5 The wall becomes the roof, the roof becomes the floor, and seamlessly, the floor becomes the roof again. The outer envelope of the structure surpasses architectural design principles in its relationship between wall and ceiling. This structure can offer stability against earthquakes and other seismic and acoustic vibrations as well as provide uniform heating and cooling inside the building.

Figure 3 Proposed Möbius car.6
Möbius Strips - Automobile
Figure 3 depicts a Möbius car designed by Tommaso Gecchelin.6 The continuously bent symmetry translates into a superb aerodynamic automotive design. This features a body that uses the concept of the Möbius strip to unify the interior and exterior in a unique and uncanny way, proving outstanding stability against collision as well as high fuel efficiency.
Möbius Strips - Belts, Recording Tape
If conveyor belts were made in the shape of Möbius strips they would wear evenly because they are flipped during each rotation, while ordinary belts wear on only one side. Similarly, recording tapes in the shape of Möbius strips may be used in devices for uninterrupted recording, potentially doubling recording and playing times.

Figure 4 Black hole model composed of a gradient-index metamaterial shell and a lossy dielectric core.10
Möbius Strips - Black Holes
A black hole is a region with a massive gravitational field that absorbs everything within its vicinity. This is due to its massive gravitational potential, influencing motion of matter propagating in a curved space-time to form a circle.7 This is similar to electromagnetic-wave propagation in a curved space or in an inhomogeneous Möbius metamaterial medium. Hence, one could use electromagnetic waves and Möbius metamaterial to mimic celestial mechanics by comparing the refractive index to the metric of gravity.8 A recently published result demonstrates the electromagnetic black hole by using metamaterials at microwave frequencies. It shows the phenomena of an electromagnetic wave bending and trapping spirally into the artificially created electromagnetic black hole.9 Cheng et al., reported a model of black hole (see Figure 4) composed of a gradient-index metamaterial shell and a lossy dielectric core that can absorb electromagnetic waves coming from all directions efficiently with an absorption rate of 99 percent.10 The absorption rate can be increased to 100 percent by incorporating a higher dimension Möbius metamaterial gradient index shell. This could yield wide applications in the thermal emitting, energy harvesting and high-Q factor evanescent mode resonators.
Möbius Strips - Graphene
Graphene has received much attention for its remarkable structural and superior electronic properties. A single layer of graphite exhibits mechanical properties like planar paper or plastic with a large bulk modulus along its plane and is easily bent or curved.11 These unique characteristics enable graphene to wrap into carbon nanotubes without deformation, making it a promising material for building Möbius metamaterial strips employed in microwave and optical components for modern communication systems. Figure 5 shows Möbius strips formed into graphene nano-ribbons that behave as topological insulators possessing topology-induced thermal and magnetic properties.11
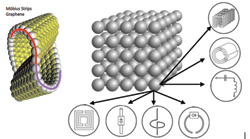
Figure 5 A typical structure of the Möbius strips formed by Graphene.11
Wang investigated the stability and total magnetic moment (TMM) of Möbius strips with fixed length and different widths.11 Möbius strips formed by Graphene nano-ribbons were found to be extraordinarily stable. These unique magnetic properties enable Möbius strip graphene-based building blocks in spintronic devices.
Möbius Strips - Passive Electrical Components
Resistors, inductors and capacitors are basic electrical components used for building electronic circuitry. In an ideal circuit, resistors would contribute only resistance, capacitors only capacitance, and inductors only inductance. This is not the case at high frequencies, however, due to unwanted electromagnetic coupling and associated parasitics.
Resistor: A resistor is an electrical component designed to reduce current flow and lower voltage in an electronic circuit. Its function as a resistor, however, is affected by parasitic reactance due to the current passing through it. A magnetic field formed on the inside and outside creates self-inductance L in series with resistance R. In addition, capacitance between turns of wire as well as between various metal objects, is equivalently represented as parasitic capacitance C.

Figure 6 Möbius strips resistor (a) and current flow direction (b).12
The L and C of a resistor cause a phase shift Ø and time constant τ at frequency ω between the current and the voltage, described by

From equation 6, L/R=RC and ω2LC,,1, the a phase shift Ø and time constant τ will tend to zero. For this reason, despite the self-inductance and parasitic capacitance associated with the resistor, a reasonably acceptable time constant can be realized at radio frequencies.
At microwave and millimeter wave frequencies, however, it is not possible to achieve ω2LC,,1; therefore, a simple resistor acts like a complex impedance. The solution to this problem is the Möbius strip resistor.12 Figure 6 shows the typical Möbius strip resistor where the inner and outer layers are connected to a terminal.
The conductive layer is a single continuous length of material having regions that are spaced from one another in the direction normal to the surface of the Möbius resistor, part of a common continuous length of conductive material affixed to the surface of a dielectric layer. The continuity of the respective dielectric and conductive layers is only in the circumferential direction running the length of the Möbius resistor, and not in the direction transverse to its length. Direct current applied at the terminal, which is between two points on the conductive surface of the Möbius resistor, will flow substantially in the circumferential direction and not in the transverse direction due to the interruption of continuity between regions of the conductive surface in the normal direction by the layer of dielectric material.
Application of an alternating voltage at the connecting point terminal induces a field in the dielectric opposing the flow of a resultant alternating current whereby the Möbius resistor exhibits a capacitive type reactance at frequencies other than those corresponding to a wavelength equal to the length of the Möbius strip or an integral multiple of its length. As a result, the Möbius resistor has the property of passing attenuated components of an applied signal having a wavelength that is not equal to the length of the Möbius strip or an integral multiple of its length.13
Consider that the strip is made of good electrical conducting material so that charges and currents flowing on the strip will end up on the boundary. From Ampere’s law, total flux ØB through the Möbius strip is given by the line integral of the currentI around the boundary ∂M. Starting at any given point on the boundary and evaluating the integral as one moves along the edge for one complete cycleof the Möbius strip M yields:
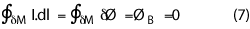
From equation 7, the total flux for the Möbius strip is zero. Using this equation, Davis12 designed and patented the Möbius resistor, where current passing through resistor’s input terminal splits into two equal pulses traveling in opposing directions due to the fact that the impedances of the two paths to the output terminal are equal. As shown in Figure 6, when the two pulses have each traveled halfway along the surface of the resistor reaching the twist (180°), they will be of equal and opposite phase, and thus will cancel. This implies net potentials tend to zero when they reach the output terminal. To achieve this result, it is necessary for the two terminals to be directly opposite, or else the pulses will not be 180° out of phase and thus will not completely cancel (implying the presence of residual magnetism). Due to the cancellation of electromagnetic fields, the Möbius resistor can be non-reactive and will maintain its properties even when folded or deformed. The characteristics of a Möbius resistor do not change with its length or form. It can be wound around a cylindrical core or a thin card, and it can even be formed into a ball, allowing it to wrap around and minimize the space it occupies.3

Figure 7 Möbius strips capacitor.13
Capacitor: The Möbius resistor has zero-residual self-inductance; however, an intrinsic negligible capacitive effect exists that can have significant utility if used for intended purposes. By modifying the material composition of the Möbius resistor from being primarily resistive to being primarily conductive, the capacitive side effect is enhanced. It is this capacitive effect of the Möbius resistor that is used by Brown13 for designing the Möbius capacitor. This structure is constructed by layering the continuous conductive surface of the typical Möbius resistor with a second dielectric material, then layering this dielectric with two separate conductive surfaces of a size compatible with the Möbius resistor. The layout of Möbius capacitor is such that the conductive surfaces are diametrically opposite to each other; while simultaneously, the normal of each conductive surface is parallel to the normal of the surface of the Möbius resistor at all points along the surface. Figure 7 shows a typical Möbius capacitor where the outer plates (P1, P2, P3 and P4) are connected via outside ribbon conductors.12
If a sinusoidal voltage v1(t) is applied at P1 then the voltage at P2 is described by

where v1(t) = VmSin ωt, Vm is the maximum amplitude, l is the length of the Möbius-resistor (if loop opened, circumference of loop equals to length of loop), u is the propagation speed between P1 and P2, f is the frequency, and t = ( ) is the wave propagation time between two opposite points P1 and P2 on the conductive surface (separated circumferentially by a distance equal to the length l of the Möbius strip).
) is the wave propagation time between two opposite points P1 and P2 on the conductive surface (separated circumferentially by a distance equal to the length l of the Möbius strip).
From equation 8, the potential difference δv across points P1 and P2 at any time t is:

The current i(t) between two normally opposite points P1 and P2 on the conductive surface of the Möbius strip as shown in Figure 6 given by the derivative of equation 8 with respect to time t:

From equation 11, if l = λ(length of Möbius strip is equal to the wavelength of field propagation) then the frequency of the wave reduces to f = , and the resulting current i(t) between two normally opposite points P1 and P2 on the conductive surface of the Möbius strip becomes zero:
, and the resulting current i(t) between two normally opposite points P1 and P2 on the conductive surface of the Möbius strip becomes zero:


Figure 8 Möbius strips filter (Möbius "twist" implemented with two vertical interconnects). Planar implementation (a) and photograph of a Möbius BPF realized on a Teflon substrate (b).15
From equation 12, when the wavelengths are equal to the length of the loop or are integer multiples, the Möbius strip in parallel with a circuit across P1 and P2 will allow a resonant frequency and its harmonics to pass through the circuit unattenuated but will attenuate other frequencies.
Resonator: The concept of the Möbius strip resonator is based on the fact that a signal coupled to a strip will not encounter any obstruction when travelling around the loop, the loop will behave like an infinite transmission line, enabling a high quality factor (Q) resonator tank. This characteristic enables many radio and microwave applications, such as a compact resonator with the resonant frequency which is half the size of identically constructed linear coils, a Tesla coil for global transmission of electricity without wires and high temperature superconductors.
Jeffrey Pond reported a Möbius strip filter (see Figure 8),14,15 in which the Möbius twist provides additional phase shift to facilitate a resonant condition and frequency selectivity in a compact size. The “twist” is implemented using two via connections through the substrate.
Möbius Strips – Metamaterial Symmetry
Symmetry is defined as a system feature or property, conserved when the system undergoes an alteration. In general, spatial symmetries are easily observed but others, such as optical symmetries in metamaterials, are concealed. Recent publications describe electromagnetic Möbius symmetries introduced into metamaterial (metamolecular), made from metals and dielectrics.16-17 The electromagnetic symmetry discovered in metamaterials is equivalent to the structural symmetry of a Möbius strip, with the number of twists controlled by the sign change of the electromagnetic coupling between the meta-atoms. The negative index artificial material (metamaterial) with different coupling signs exhibit resonant frequencies that depend only on the number of turns but not the locations of the ‘‘twists,’’ thus confirming its Möbius symmetry topological nature.
Figure 9 illustrates the hyperspace Möbius mechanism that transforms ordinary benzene molecules into metamolecules with Möbius symmetry (the topological phenomenon that yields a half-twisted strip with two surfaces but only one side).16 Figure 9 shows a three-body system like a trimmer with metallic resonant meta-atoms configured as coupled split-ring resonators, symbolized as metamolecules exhibiting topological Möbius-cyclic-symmetry (C3-symmetry) through three rotations of 120°. Möbius twists result from a change in the signs of the electromagnetic coupling constants between the constituent meta-atoms.17
Conclusion
Since its discovery, the Möbius strip has captured the imaginations of mathematicians and engineers everywhere, and it continues to fascinate modern generations with its non-orientability and one-sidedness. The unique features of Möbius strips promise new inventions, recurring in an endless loop; one might say that the future resides on the surface of Möbius strips.

Figure 9 Möbius symmetry in metamolecular trimmers made from metals and dielectrics (courtesy: National Science Foundation).17
Note: This article will be continued with Part II (Möbius Metamaterial Resonator) and Part III (Möbius Metamaterial Oscillators) in the December 2014 and January 2015 issues, respectively.18
References
- Hückel, Grundzüge der Theorie ungesättiger und aromatischer Verbindungen, Verlag Chem, Berlin, 1938, pp. 77-85.
- E.L. Starostin and G.H.M. van der Heijden, “The Shape of a Möbius Strip,” Nature Materials, Vol. 6, July 2007, pp. 563-567.
- C.A. Pickover, The Möbius Strip: Dr. August Möbius’s Marvelous Band in Mathematics, Games, Literature, Art, Technology, and Cosmology, Thunder’s Mouth Press, New York, N.Y., 2006.
- “Möbius Strip Ties Liquid Crystal in Knots,” R&D Magazine, August 20, 2013, www.rdmag.com/news/2013/08/m%C3%B6bius-strip-ties-liquid-crystal-knots, accessed September 2014.
- D. Basulto, “National Library in Astana, Kazakhstan / BIG,” ArchDaily, August 25, 2009, www.archdaily.com/33238/national-library-in-astana-kazakhstan-big/, accessed September 2014.
- T. Gecchelin, “Möbius Concept Car Design,” Automotive Design, Engineering, Industrial Design, 2010, http://tommasogecchelin.prosite.com/208236/812241/gallery/Möbius-concept-car-design, accessed September 2014.
- A.J. Kox, M.J. Klein and R. Schulmann Kox, The Collected Papers of Albert Einstein, Vol. 6, Princeton University Press, 1997.
- D.A. Genov, S. Zhang and X. Zhang, “Mimicking Celestial Mechanics in Metamaterials,” Nature Physics, Vol. 5, July 2009, pp. 687-692.
- E.E. Narimanov and A.V. Kildishev, “Optical Black Hole: Broadband Omni-Directional Light Absorber,” Applied Physics Letters, Vol. 95, July 2009.
- Q. Cheng, T.J. Cui, W.X. Jiang and B.G. Cai, “An Electromagnetic Black Hole Made of Metamaterials, Cornell University Library, April 30, 2010, http://arxiv.org/abs/0910.2159, accessed September 2014.
- X. Wang, X.H. Zheng, M. Ni, L. Zou and Z. Zeng,“Theoretical Investigation of Mobous Strips Formed From Graphene,”Applied Physics Letters, Vol. 97, No. 12, September 2010, pp. 123103 - 123103-3.
- R.L. Davis, “Non-Inductive Electrical Resistor,” US Patent 3267406A, August 16, 1966.
- T.J. Brown, “Möbius Capacitor,” US Patent 4599586, July 8, 1986.
- J.M. Pond, “Möbius Resonator and Filter,” US Patent 6445264, September 3, 2002.
- J.M. Pond, “Möbius Dual Mode Resonators and Bandpass Filters,” IEEE Transactions on Microwave Theory and Techniques, Vol. 48, No. 12, December 2000, pp. 2465-2471.
- C.W. Chang, M. Liu, S. Nam, S. Zhang, Y. Liu, G. Bartal and X. Zhang,” Optical Möbius Symmetry in Metamaterials,” Physical Review Letters, Vol. 105, December 2010, pp. 235501-1-235501-4.
- Lynn Yarris, “Strange New Twist: Berkeley Researchers Discover Möbius Symmetry in Metamaterials,” Berkeley Lab, 20 December 2010, http://newscenter.lbl.gov/2010/12/20/Möbius-symmetry-in-metamaterials/#sthash.Ezqlm5Pr.dpuf, accessed September 2014.
- A.K. Poddar, “Slow-Wave Resonator Based Tunable Multi-Band Multi-Mode Injection-Locked Oscillators,” Research Report, RF and Microwave Techniques Brandenburg University of Technology Cottbus-Senftenberg 2014,” https://www-docs.tu-cottbus.de/mikrowellentechnik/public/poddar_report_long_ebook.pdf.
