All quartz crystal oscillators are affected by acceleration. While for many applications the effects are negligible, in those where frequency stability is critical and the environment is physically demanding (high shock or vibration), the effects can be significant. Even devices that are operated in relatively benign locations are under the influence of gravity and possibly low level vibration. If they are rotated or moved, a small shift in the operating frequency will occur. Figure 1 shows a precision quartz resonator mounted in a four-point holder.


The acceleration sensitivity characteristic of a quartz crystal, commonly referred to as Gamma (), is vectorial in nature. 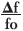The frequency shift that will be exhibited by a crystal experiencing an acceleration is therefore dependent on the direction and magnitude of the applied force as well as the magnitude and orientation of the inherent acceleration or “g-sensitivity” vector.1 The fractional frequency change of the oscillator under an acceleration is given by the inner product of and , that is

Figure 1 Four-point mount crystal blank.

The acceleration sensitivity vector can be determined by measuring the frequency change under acceleration in three linearly independent directions (e.g. three orthogonal directions) that are aligned with the faces of the oscillator or crystal package. A frame of reference is therefore defined. The magnitude || of is given by the square-root of the sum of the squares of its components (Γx, Γy, Γz)

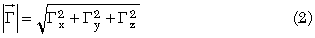
Figure 2 Relative frequency shift as a result of applied acceleration.

Figure 3 Gamma vector measurement frame.

It can be seen that the fractional frequency change is maximally positive when is parallel to ; it is maximally negative when is antiparallel to , and approaches zero when is perpendicular to. This relationship is illustrated in Figure 2. The direction of relative to the measurement frame as shown in Figure 3 may then be determined.4

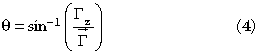
Normally the dominant source of the oscillator’s acceleration sensitivity is the quartz crystal resonator. Depending on the construction, a given group of crystals may exhibit substantial variation in both the direction and magnitude of . It is therefore necessary to measure each crystal in order to be assured of its individual characteristic.

The acceleration sensitivity of a quartz crystal is caused primarily by non-uniform stresses induced within the active area of the quartz blank by the acceleration. The active area of a crystal resonator is in the center of the blank, between the plated electrodes. Conventional crystals are typically mounted at two points in a flat holder such as an HC-43 or HC-45. Round holders such as the HC-35 or HC-37 (TO-05 or TO-08 size) mount the crystal horizontally at three or four points. The resonant frequency of any mechanical body is inherently sensitive to stresses and strains that cause the resonant frequency to change. An applied acceleration in any direction will impart a stress to the quartz that is non-uniform in some manner due to the mounts. Typical crystal acceleration sensitivities will range from about 1 x 10-10 per g for specially constructed precision SC-cut and AT-cut resonators to the order of 10-7 per g for tuning fork resonators.2

Figure 4 Internal view of crystal strip mount.

With the drive to continually reduce the package size of crystal oscillators for new systems and applications, improvements have been made in the performance of miniature strip crystals. These resonators use small rectangular quartz blanks instead of the round wafers found in conventional holders, as shown in Figure 4.5 Although these crystals have been used for some time in low cost oscillators, recent advances in their design and processing have provided resonators for some precision applications that are just as good or even better in some respects than the much larger conventional holders. The blanks are mounted at one end and suspended in a cantilever fashion. The low mass of the quartz and the isolation of the active area from the stress of the mount can provide a design with low acceleration sensitivity with some being better than 1x10-9 per g.

Environmental Effects

The type of acceleration that the crystal is exposed to will determine how the frequency will respond. It is common for an oscillator to experience mechanical shocks due to routine handling of equipment or movements in the vicinity of the oscillator. These types of shocks will cause a temporary perturbation in the frequency that could disturb the operation of circuitry such as narrowband phase-locked loops. Reducing these effects requires either attenuation of the applied shock or improving the acceleration sensitivity of the oscillator.

Oscillators that are deployed in a mobile environment such as a vehicular application may experience significant levels of vibration. Through the acceleration sensitivity of the oscillator, this vibration modulates the output frequency. Random vibration on some airborne platforms can reach extremely high levels. This wideband mechanical vibration leads to wideband phase noise degradation in the oscillator. Periodic vibrations from an engine, rotating machinery or even a cooling fan can induce discrete sidebands on the output signal. The levels of the sidebands will be determined by the magnitude and frequency of the vibration as well as the acceleration sensitivity component of the crystal in the direction of the force.1

Another influence that affects all oscillators is the constant acceleration due to gravity. All bodies at rest on the surface of the earth experience an acceleration of 1 g directed upward. Rotating an oscillator around a horizontal axis will change the angle between and . Through Equation 1 it can be seen that a frequency change will occur. For many applications this change is negligible, but for those requiring stability on the order of 1 ppb or better, this amount of frequency shift can be significant.

Acceleration Sensitivity Measurement Methods

There are several methods that can be used to measure the acceleration sensitivity of an oscillator. Here we discuss the simple 2-g tipover test and the vibration induced sideband methods.

A technique that uses the constant gravitational acceleration of the earth to cause a measurable frequency shift in an oscillator is known as the “2-g tipover test”. A set of orthogonal axes relative to the oscillator case is defined. The oscillator is oriented so that one axis (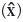) points upwards and the frequency is measured. The oscillator is then rotated 180° so that this axis points downwards and the frequency is measured in this orientation. From the point of view of the oscillator, this is as though the acceleration changed from

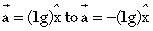
so the resultant frequency difference between the two configurations would be given by

There is a net 2 g change in the acceleration so x is one half of the fractional frequency change (in units per g). Repeating this for the other two axes gives all three components of and hence is defined. This method requires a relatively stable oscillator in order to see the gravitational induced shifts above the short-term noise and thermal drift of the oscillator. Several cycles may be needed to separate the g sensitivity from these effects.

Figure 5 Oscillator spectrum under sine vibration.

An accurate method for measuring the acceleration sensitivity of any oscillator uses mechanical vibration to modulate the output frequency of the oscillator through the acceleration sensitivity effect (see Figure 5). The effect on the output signal can then be observed with a spectrum analyzer. Sinusoidal vibrations are used to characterize the sensitivity at a specific frequency. Under sinusoidal vibration, discrete sidebands will be induced on the oscillator output signal offset from the carrier ƒo by the vibration frequency ƒv. Since the sinusoidal vibration essentially FM modulates the carrier, the level of the sidebands will be predicted by FM modulation theory. For a signal whose frequency has a peak change of Δƒ at a modulating frequency ƒm, the measured sideband levels at frequency ƒo±ƒm will have good approximation to the modulation index formula for a small index of <0.1.2

The amount of frequency shift is given by Δƒ=|⋅|ƒ0 and the modulation frequency is the frequency of the sinusoidal vibration ƒv so that the formula for the level of the vibration induced sidebands becomes:

By rearranging the formula, the measured sideband level in dBc can be converted to the corresponding g-sensitivity in the direction being vibrated, d, given the known vibration g-level αd and nominal frequency ƒo.

The acceleration sensitivity is fairly constant with frequency as long as the vibration frequency is below the lowest mechanical mode of the resonator. This could be as high as two or three kHz for a strip crystal or a stiff four-point mount or as low as 100 Hz for a large crystal using a spring type mount. The frequency shift is also linearly proportional to the applied g level up to about 50 g’s. For screening purposes a vibration frequency and acceleration level are chosen that give easily measurable sidebands.

Effects of Random Vibration

Figure 6 Phase noise degradation due to random vibration.

Under random vibrations the oscillator will experience accelerations over a wide range of frequencies and various magnitudes. Even a moderate amount of random vibration can cause a significant degradation of an oscillator’s phase noise performance (see Figure 6). Similar modulation index arguments are used to calculate the vibration induced noise at a frequency offset, fr. These random motions are characterized by the power spectral density of the vibration profile. The formula for approximating the induced noise at offset fr becomes:

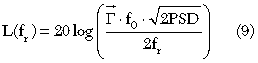
When the phase noise of the oscillator is measured while vibrating, it is then possible to calculate the g-sensitivity at any frequency within the spectrum. Care must be taken to ensure that fixture resonances and cable sensitivities are accounted for when evaluating the results.

Screening for Acceleration Sensitivity

There are some applications where the performance of the crystal oscillator under vibration or shock is a critical parameter that must be guaranteed 100 percent to a stringent level. Although robust crystal designs and well controlled production processes can produce crystals with high yields, screening is still necessary to ensure some specifications. A typical screening system would consist of a vibration table and controller, fixturing for securing the crystals in an oscillator and a narrow-band spectrum analyzer or other instrument for evaluating the levels of vibration induced sidebands. A vibration level and frequency are selected that will produce measureable sideband levels, but are convenient to work with. A level of 10 g’s at a frequency of 90 Hz is a typical choice. Figure 7 shows the results of screening a group of 20 MHz TCXOs that use AT strip crystals.

Figure 7 Screening results for a group of 20 MHz TCXOs.

Methods for Reducing Sensitivity

There are methods and techniques that can be employed to improve the acceleration performance of a crystal oscillator. For example, if the acceleration sensitivity vector is reasonably consistent in direction and if the accelerations occur primarily in one direction, then aligning the oscillator so that the accelerations are perpendicular to can result in as much as a 10-fold reduction in frequency changes compared to a worst case alignment.

Active acceleration compensation may be implemented by utilizing an accelerometer with feedback to the oscillator circuit. This closed loop system is then accurately calibrated to offset the frequency of the oscillator proportional to the sensed acceleration and cancel out the frequency shifts caused by the acceleration. This technique can work well at lower frequencies up through a few hundred Hz, but it requires a relatively complex circuit to sense acceleration in all three axes, scale the correction signal properly and account for frequency dependent phase shifts in the circuitry. Close attention to the mechanical details of the design is also required.

Another type of compensation involves using multiple crystals with their acceleration sensitivity vectors aligned so that they are pointing in opposite directions in an anti-parallel arrangement.3 The crystals are then connected to the oscillator circuit either in a parallel or series configuration where the composite combination operates as a single crystal with modified motional parameters. The two vectors then essentially cancel the effect of each other. This method can be quite effective if the crystals are similar and are characterized and aligned properly in the assembly. With a solid mechanical design, cancellation will occur up through several kHz and can provide a significant improvement in phase noise performance during random vibration.

Some systems designed for severe environments or very sensitive installations can effectively use vibration and shock isolators to mechanically attenuate the force that is transmitted to the oscillator. Vibration isolators are only effective in reducing levels above the natural resonant frequency of the system. Obtaining a low resonant frequency below a few hundred Hz is difficult with a small, light component such as a miniature oscillator and it may be necessary to add weight to the assembly depending on the stiffness of the isolator. There is a region near the resonant frequency where the force is actually amplified by some amount depending on the damping characteristics of the materials. Care must be taken to ensure sufficient sway room to allow for movement of the assembly, especially if excited near the resonant frequency. An isolation system that is not properly designed can make the overall performance worse rather than better.

While acceleration forces are unavoidable, by understanding their effects on oscillator performance and the methods that may be used to mitigate these effects, acceptable performance can be achieved in most application environments. Advancements in crystal technology continue, but the “zero g-sensitivity” oscillator is not yet a reality so dealing with the effects of acceleration will be necessary for the foreseeable future.

References

  1. R.L. Filler, “The Acceleration Sensitivity of Quartz Crystal Oscillators: A Review,” IEEE Transactions on Ultrasonics, Ferroelectrics and Frequency Control, Vol. 35, No. 3, May 1988, pp. 297-305.
  2. J.R. Vig, “Quartz Crystal Resonators and Oscillators for Frequency Control and Timing Applications: A Tutorial,” Rev. 8.5.3.0, February, 2005, AD-M001251(revised).
  3. R.L. Filler, “Acceleration Resistant Crystal Resonator,” U.S. Patent No. 4,410,822, 1983.
  4. J.M. Przyjemski, “Improvement in System Performance Using a Crystal Oscillator Compensated for Acceleration Sensitivity,” Proc. 32nd Annual Frequency Control Symposium, pp. 426-431, 1978.
  5. Statek Corp., Technical Note 28, “An Ultra-miniature Low Profile at Quartz Resonator.”